Tag Archives: scheme

“Locally of finite type” is a local property

Posted on by

0

Previously we showed that morphisms locally of finite type are preserved under base change. We can use this to show that

(*) Given a morphism of schemes p: X \to Y, the preimage of any affine \text{Spec }A \subset Y can be covered by affines such that the corresponding ring maps are of finite type.

Alternatively, if we define a morphism locally of finite type to be one that satisfies (*), then what we are saying is that such a property can be checked on a cover; we can replace “any affine” with “an affine in a cover of affines”.

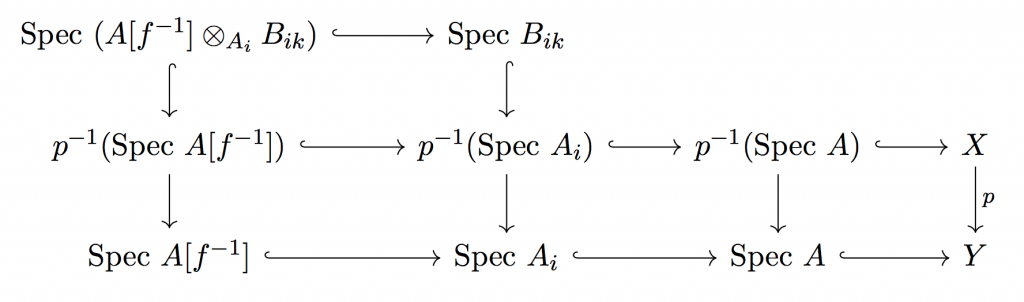
Let’s try to prove (*). First, we base change to \text{Spec }A. Since the morphism p^{-1}(\text{Spec }A) \to \text{Spec }A is also locally of finite type, we can cover \text{Spec }A by affines \text{Spec }A_i such that their preimages can be covered by the spectra of finitely-generated A_i-algebras B_{ik}. However, we don’t know if these are finitely-generated A-algebras! To fix this, we base change to even smaller affines. Cover \text{Spec }A_i by basic open sets \text{Spec }A[f^{-1}]. This gives us a cover of each \text{Spec }B_{ik} by basic open sets of the form \text{Spec }(A[f^{-1}] \otimes_{A_i} B_{ik}). Since A_i \to B_{ik} is of finite type, A[f^{-1}] \to A[f^{-1}] \otimes_{A_i} B_{ik} is of finite type. Since A \to A[f^{-1}] is clearly of finite type, A \to A[f^{-1}] \otimes_{A_i} B_{ik} is of finite type, giving us the desired cover of p^{-1}(\text{Spec }A). 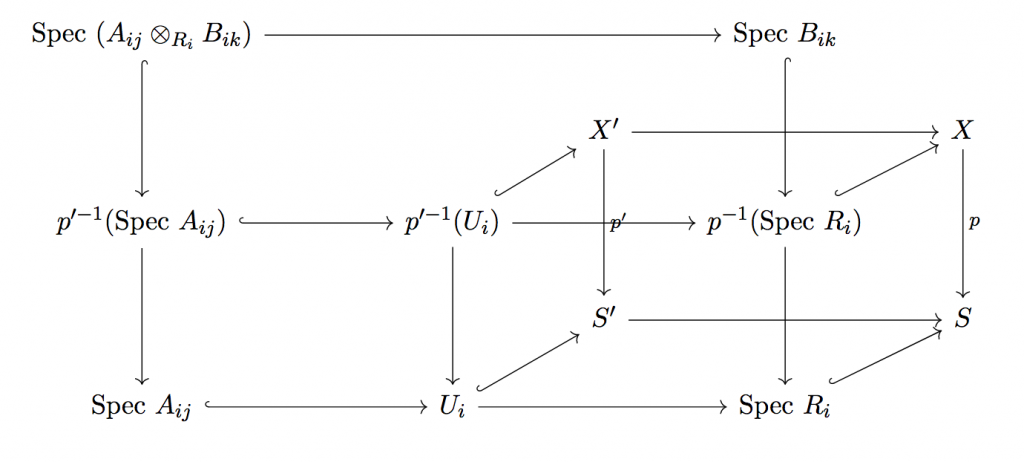The following diagram may be illustrative (every square is a pullback)

Group schemes and graded rings

Posted on by

0

In this post we will describe how an action of the multiplicative group scheme \mathbb{G}_m on \text{Spec }R defines a \mathbb{Z}-grading of R. A future post may describe how this relates to projective schemes. (I will do all of this using diagrams, but there may be some easier way using the functors of points). All this was taught to me by Mark Haiman in Math 256B (Algebraic Geometry) at UC Berkeley.

Fix a field k; we will work in the category of k-schemes. Thus R will be a k-algebra, and we will establish a graded k-algebra structure on R. However, none of our arguments change if we just let k be \mathbb{Z}. A group scheme is a group object in the category of k-schemes. A precise definition can be found here. Most importantly, group schemes can act on other schemes. The definition of a group scheme action can be found here. Note that all definitions are given by diagrams (or functor of points). For example, we specify the “identity element” of a group scheme by a map \text{Spec }k \to G, rather than selecting some point in the underlying topological space.

\mathbb{G}_m is defined as \text{Spec }k[s, t]/(st-1) = \text{Spec }k[t, t^{-1}]. (for shorthand, we will write k[t, t^{-1}] as k[t^\pm]). As a variety, it can be thought of as k^*, the “punctured affine line”. Its group operation is given by a map \mathbb{G}_m \times_k \mathbb{G}_m \to \mathbb{G}_m which corresponds to the k-algebra map \mu: k[t^\pm] \to k[t^\pm] \otimes_k k[t^\pm] \cong k[t^\pm, u^\pm] defined by t \mapsto tu. The identity is given by a map \text{Spec }k \to \mathbb{G}_m corresponding to i: k[t^\pm] \to k defined by t \mapsto 1.

Suppose \mathbb{G}_m acts on \Spec R. The action map \mathbb{G}_m \times_k \text{Spec }R \to \text{Spec }R corresponds to a k-algebra map \phi: R \to R \otimes_k k[t^\pm] \cong R[t^\pm] such that the following diagrams commute:

Associativity:

\xymatrix{R\ar[r]^{\phi}\ar[d]^{\phi} & {R[t^\pm]} \ar[d]^{id_R\otimes \mu}\\{R[u^\pm]} \ar[r]^{\phi \otimes id_{k[u^\pm]}} & {R[t^\pm, u^\pm]}}

Identity:

\xymatrix{R\ar[r]^{\phi}\ar[d]^{id_R} & {R[t^\pm]} \ar[dl]^{i}\\ R}

For r \in R, write \phi(r) = \sum_{-\infty}^{\infty} r_it^i \in R[t^\pm], where almost all the r_i are zero. Then the first diagram implies that

(*) if \phi(r) = r_it^i (i.e. the polynomial is just a single monomial), then \phi(r_i) = r_it^i.

This is because, along the top and right arrows, we have r \mapsto r_it^i \mapsto r_it^iu^i and along the left and bottom arrows we have r \mapsto r_iu^i \mapsto \phi(r_i)u^i. Furthermore, the second diagram says that

(**) for all r, \sum r_i = r.

Therefore, letting R[t^\pm]_d stand for the degree d homogenous component of R[t^\pm] (so that it consists of multiples of t^d), let R_d := \phi^{-1}(R[t^\pm]_d). Since all the R[t^\pm]_d are disjoint, their preimages are disjoint as well. Furthermore, for an arbitrary element r, we have r = \sum r_i by (*), and by (**), we have that each r_i \in R_i. Thus R = \sum R_i as a direct sum.

It remains to show that R_mR_n \subseteq R_{m+n}. But this is easy: if r_m \in R_m, r_n \in R_n, then \phi(r_mr_n) = \phi(r_m)\phi(r_n) = r_mr_nx^{m+n} \in R[t^\pm]_{m+n}, so r_mr_n \in R_{m+n} as desired.