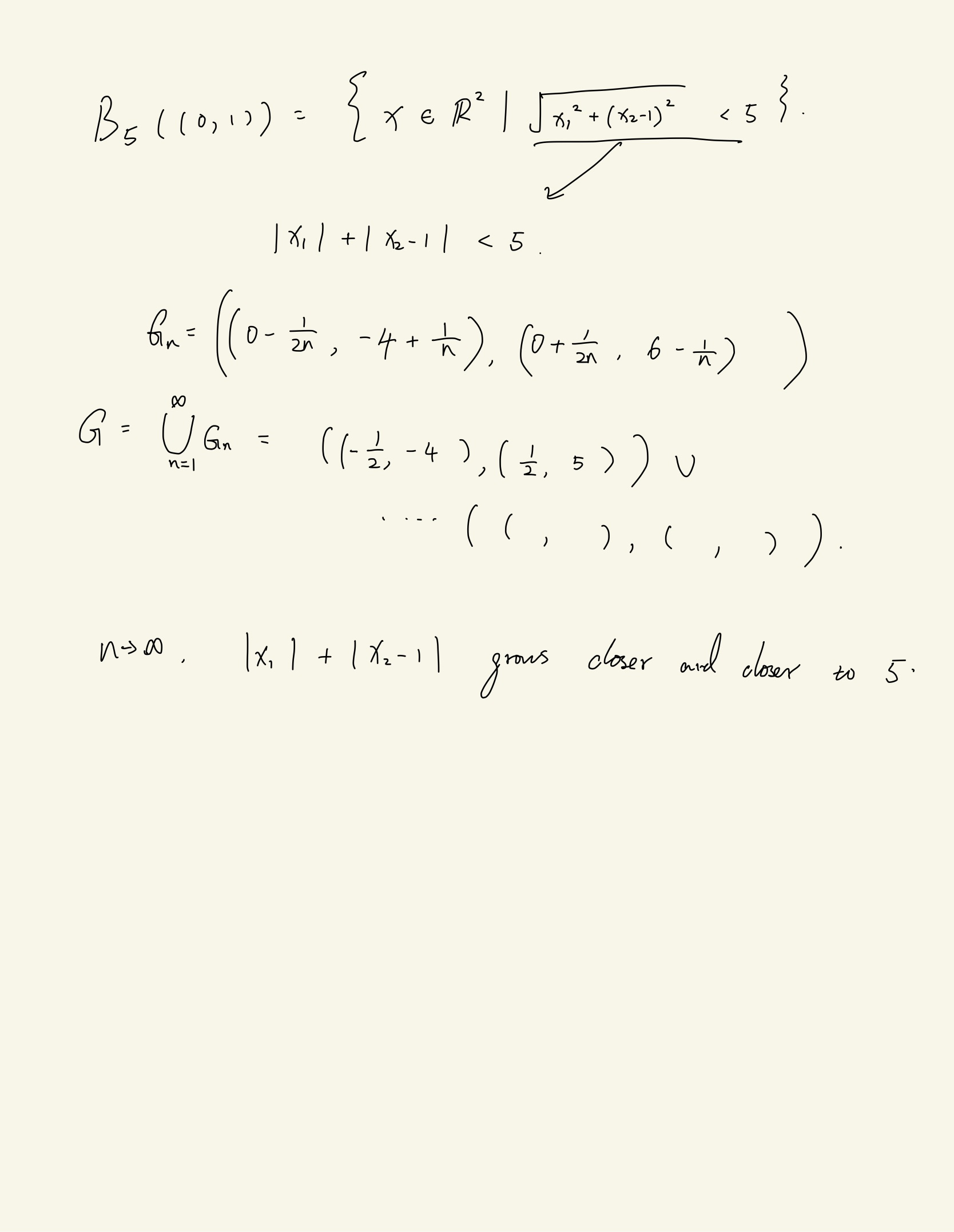Questions:

1. From the lecture on April 15th
   1. 
   • I cannot think of why $x^2$ is an example of f(x) ≠ P_x0(x)
   • I tried fixing $x_0 = 0$, so $P_0(x) = ∑ c_n \cdot (x)^n$, where $\alpha = lim sup |c_n|^{1/n} = 1$, so $R=1$
   • Then $f(0.5) = 0.25 = P_0(0.5) = 0 + 0 + 1 * 0.5 ^ 2 + 0 + … + 0$
2. A question from my friend, finding open cover
   1. 
   2. My attempt:
   •