Author |
Topic: New conjecture (Read 8011 times) |
|
aicoped
Junior Member
Gender: 
Posts: 57
|
 |
New conjecture
« on: Sep 11th, 2011, 5:20pm » |
 Quote  Modify
|
Ok when I was a young lad I was playing around with squares and cubes and whatnot one summer and I discovered and figured out the formula for what i later learned was already called Waring's conjecture.
Briefly for every power there exists a maximal number such that all positive integers can be expressed as the sum of that many or less terms to the given power.
For squares, every positive integer can be expressed as the sum of 4 or less square numbers.
Cubes take 9.
Fourth powers take no more than 19 and so on.
Now here is the interesting thing to me at least. Once numbers start getting relatively large their maximal number for that power goes down(except for squares, which possibly always will always need 4 for every number of the form 8x-1).
With all that being said, here is my conjecture. To my knowledge it is unique to me. if anyone can show me a proof or direct me to someone that conjectured it already, I would be appreciative.
Any sufficiently large number can be expressed as the sum of at most 3 positive integers taken to powers 2 or higher(these powers need not be the same).
for example 127 takes 4 squares to do(11^2+2^2+1^2+1^2), but only 2 numbers if multiple powers are allowed(10^2+3^3).
|
|
 IP Logged |
|
|
|
william wu
wu::riddles Administrator
Gender:
Posts: 1291
|
|
Re: New conjecture
« Reply #1 on: Jan 22nd, 2012, 1:49pm » |
Quote Modify
|
Usually when I see a conjecture like this, I also want to see a computer printout of some sort --- perhaps generated by a brute force program --- that shows that the conjecture is true for many many numbers before anyone bothers trying to prove that it is true. Do you have such evidence?
|
| « Last Edit: Jan 22nd, 2012, 1:49pm by william wu » |
IP Logged |
[ wu ] : http://wuriddles.com / http://forums.wuriddles.com
|
|
|
SMQ
wu::riddles Moderator
Uberpuzzler
Gender:
Posts: 2084
|
|
Re: New conjecture
« Reply #2 on: Feb 13th, 2012, 2:06pm » |
Quote Modify
|
I can currently confirm by computer search that the conjecture holds for 1  n 4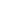 1010, but I'm not yet confident it holds generally.
Also note that the conjecture can equivalently be stated as "all positive integers of the form 4s(8t + 7) can be represented as the sum of three perfect powers of non-negative integers" since it is known that all positive integers not of the form 4s(8t + 7) can be represented as the sum of three squares. (Gauss, after an near-proof by Legendre).
--SMQ
|
|
IP Logged |
--SMQ
|
|
|
|
RIDDLES SITE
WRITE MATH!
Home 
Help 
Search 
Members 
Login 
Register
Reply
Notify of replies
Send Topic
Print