 Author
Author |
Topic: Extending x^x (Read 987 times) |
|
Icarus
wu::riddles Moderator
Uberpuzzler
    

Boldly going where even angels fear to tread.
Gender: 
Posts: 4863
|
Oddly enough, this question was not inspired by other appearances of xx lately:
If we restrict ourselves to the real numbers, the function f(x) = xx is generally considered for x >=0 only. However values can also be defined for x < 0 when x is a rational number with odd denominator (in lowest terms). If we restrict the numerator to evens, the values of xx are even positive.
Since rationals with even numerators and odd denominators are dense, we can use this to extend f(x) to the entire real line. In particular, let D = { 2p/q : gcd(2p,q) = 1}, and consider xx defined on D. For all t  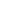 , define f(t) = limx , define f(t) = limx t; x t; x D xx. D xx.
How differentiable is f? I.e., for what values of k is f  Ck? Is f analytic? Ck? Is f analytic?
|
|
 IP Logged IP Logged |
"Pi goes on and on and on ...
And e is just as cursed.
I wonder: Which is larger
When their digits are reversed? " - Anonymous
|
|
|
Obob
Senior Riddler
   

Gender: 
Posts: 489
|
 |
Re: Extending x^x
« Reply #1 on: Feb 10th, 2008, 11:42am » |
 Quote Quote  Modify Modify
|
For all x in D, we have xx=|x|x since the numerator of x is even. But |x|x is defined and continuous on the whole negative real axis, so f=|x|x. Clearly then f is infinitely differentiable on the whole negative real axis.
In fact, f is analytic. For on the negative real axis we can write |x|x=(-x)x. The function (-z)z is a multi-valued analytic function on the punctured complex plane C-{0} which is single-valued on the negative real axis and coincides with f there.
|
|
 IP Logged IP Logged |
|
|
|
temporary
Full Member
  

Posts: 255
|
 |
Re: Extending x^x
« Reply #2 on: Feb 10th, 2008, 12:22pm » |
 Quote Quote  Modify Modify
|
on Feb 9th, 2008, 4:38pm, Icarus wrote:Oddly enough, this question was not inspired by other appearances of xx lately:
If we restrict ourselves to the real numbers, the function f(x) = xx is generally considered for x >=0 only. However values can also be defined for x < 0 when x is a rational number with odd denominator (in lowest terms). If we restrict the numerator to evens, the values of xx are even positive.
Since rationals with even numerators and odd denominators are dense, we can use this to extend f(x) to the entire real line. In particular, let D = { 2p/q : gcd(2p,q) = 1}, and consider xx defined on D. For all t  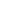 , define f(t) = limx , define f(t) = limx t; x t; x D xx. D xx.
How differentiable is f? I.e., for what values of k is f  Ck? Is f analytic? Ck? Is f analytic? |
|
Since when is f(x)=x^x only defined to x>=0?
|
|
 IP Logged IP Logged |
My goal is to find what my goal is, once I find what my goal is, my goal will be complete.
|
|
|
Icarus
wu::riddles Moderator
Uberpuzzler
    

Boldly going where even angels fear to tread.
Gender: 
Posts: 4863
|
 |
Re: Extending x^x
« Reply #3 on: Feb 10th, 2008, 12:47pm » |
 Quote Quote  Modify Modify
|
You might want to try some reading comprehension courses, temporary. You don't seem to be very good at it. I didn't say that x^x was only defined for x > 0. In fact a part of what I said is that it can be defined for some x < 0.
|
| « Last Edit: Feb 10th, 2008, 12:50pm by Icarus » |
 IP Logged IP Logged |
"Pi goes on and on and on ...
And e is just as cursed.
I wonder: Which is larger
When their digits are reversed? " - Anonymous
|
|
|
Icarus
wu::riddles Moderator
Uberpuzzler
    

Boldly going where even angels fear to tread.
Gender: 
Posts: 4863
|
 |
Re: Extending x^x
« Reply #4 on: Feb 10th, 2008, 12:49pm » |
 Quote Quote  Modify Modify
|
on Feb 10th, 2008, 11:42am, Obob wrote:For all x in D, we have xx=|x|x since the numerator of x is even. But |x|x is defined and continuous on the whole negative real axis, so f=|x|x. Clearly then f is infinitely differentiable on the whole negative real axis.
In fact, f is analytic. For on the negative real axis we can write |x|x=(-x)x. The function (-z)z is a multi-valued analytic function on the punctured complex plane C-{0} which is single-valued on the negative real axis and coincides with f there. |
|
I seem to be overly complicating things lately. I should have put this in Easy, not Putnam!
|
|
 IP Logged IP Logged |
"Pi goes on and on and on ...
And e is just as cursed.
I wonder: Which is larger
When their digits are reversed? " - Anonymous
|
|
|
Obob
Senior Riddler
   

Gender: 
Posts: 489
|
 |
Re: Extending x^x
« Reply #5 on: Feb 10th, 2008, 1:29pm » |
 Quote Quote  Modify Modify
|
I suppose I did gloss over one point: f is not differentiable at 0. But this shouldn't be surprising, since limx->0+ d(xx)/dx = -infty.
|
|
 IP Logged IP Logged |
|
|
|
|
 WRITE MATH!
WRITE MATH!
 Home
Home  Help
Help  Search
Search  Members
Members  Login
Login  Register
Register WRITE MATH!
WRITE MATH!
 Home
Home  Help
Help  Search
Search  Members
Members  Login
Login  Register
Register