 Author
Author |
Topic: x^x=5 (Read 5630 times) |
|
temporary
Full Member
  

Posts: 255
|
The title says it all. Try x^x=i if you have already solved the previous one. Hintsqrt(n)^sqrt(n)^sqrt(n)...=n when done from up to down
|
| « Last Edit: Jan 23rd, 2008, 6:40pm by temporary » |
 IP Logged IP Logged |
My goal is to find what my goal is, once I find what my goal is, my goal will be complete.
|
|
|
Obob
Senior Riddler
   

Gender: 
Posts: 489
|
 |
Re: x^x=5
« Reply #1 on: Jan 23rd, 2008, 11:39pm » |
 Quote Quote  Modify Modify
|
Please don't post riddles when you have no idea what you are talking about.
|
|
 IP Logged IP Logged |
|
|
|
Michael Dagg
Senior Riddler
   

Gender: 
Posts: 500
|
 |
Re: x^x=5
« Reply #2 on: Jan 24th, 2008, 12:20am » |
 Quote Quote  Modify Modify
|
Thinking sensibly, you can contrive an exponential
function for this equation that is a Lambert function
-- not really difficult either.
|
|
 IP Logged IP Logged |
Regards,
Michael Dagg
|
|
|
Icarus
wu::riddles Moderator
Uberpuzzler
    

Boldly going where even angels fear to tread.
Gender: 
Posts: 4863
|
 |
Re: x^x=5
« Reply #4 on: Jan 24th, 2008, 4:56pm » |
 Quote Quote  Modify Modify
|
The "hint" is easily demonstrated to be false. If it were true, then ( n)n = n. But this is not true for most values of n. n)n = n. But this is not true for most values of n.
That ii = i is a fun little result. iz is normally a multi-valued function. But all the various values collapse at z = i to just one.
As for xx = 5, I get x = 2.129372483...
|
|
 IP Logged IP Logged |
"Pi goes on and on and on ...
And e is just as cursed.
I wonder: Which is larger
When their digits are reversed? " - Anonymous
|
|
|
ThudnBlunder
Uberpuzzler
    

The dewdrop slides into the shining Sea
Gender: 
Posts: 4489
|
 |
Re: x^x=5
« Reply #5 on: Jan 24th, 2008, 6:20pm » |
 Quote Quote  Modify Modify
|
on Jan 24th, 2008, 4:56pm, Icarus wrote:
That ii = i is a fun little result.
|
|
I would call that funny. 
|
|
 IP Logged IP Logged |
THE MEEK SHALL INHERIT THE EARTH.....................................................................er, if that's all right with the rest of you.
|
|
|
Obob
Senior Riddler
   

Gender: 
Posts: 489
|
 |
Re: x^x=5
« Reply #6 on: Jan 24th, 2008, 6:25pm » |
 Quote Quote  Modify Modify
|
Unfortunately ii=i also isn't true... It is true that ii isn't multi-valued, but the value is e- pi/2.
|
|
 IP Logged IP Logged |
|
|
|
Icarus
wu::riddles Moderator
Uberpuzzler
    

Boldly going where even angels fear to tread.
Gender: 
Posts: 4863
|
 |
Re: x^x=5
« Reply #7 on: Jan 24th, 2008, 6:35pm » |
 Quote Quote  Modify Modify
|
&$#*(&!!  
Dropped an i and didn't notice it!
|
|
 IP Logged IP Logged |
"Pi goes on and on and on ...
And e is just as cursed.
I wonder: Which is larger
When their digits are reversed? " - Anonymous
|
|
|
temporary
Full Member
  

Posts: 255
|
 |
Re: x^x=5
« Reply #8 on: Jan 24th, 2008, 7:52pm » |
 Quote Quote  Modify Modify
|
I don't know where you get i^i=i, but i^i=e^-pi/2 +-2pi(k) where k is any integer.
|
| « Last Edit: Jan 24th, 2008, 7:53pm by temporary » |
 IP Logged IP Logged |
My goal is to find what my goal is, once I find what my goal is, my goal will be complete.
|
|
|
Icarus
wu::riddles Moderator
Uberpuzzler
    

Boldly going where even angels fear to tread.
Gender: 
Posts: 4863
|
 |
Re: x^x=5
« Reply #9 on: Jan 24th, 2008, 8:29pm » |
 Quote Quote  Modify Modify
|
You might consider reading the three posts following that one, where this little mystery is solved.
Of course, I have the small satisfaction of partially confusing Obob with my gibberish. You are correct that ii is multivalued.
|
|
 IP Logged IP Logged |
"Pi goes on and on and on ...
And e is just as cursed.
I wonder: Which is larger
When their digits are reversed? " - Anonymous
|
|
|
temporary
Full Member
  

Posts: 255
|
 |
Re: x^x=5
« Reply #10 on: Jan 24th, 2008, 9:14pm » |
 Quote Quote  Modify Modify
|
Especially since ^i involves natural logarithm, which is endlessly multivalued.
|
|
 IP Logged IP Logged |
My goal is to find what my goal is, once I find what my goal is, my goal will be complete.
|
|
|
Obob
Senior Riddler
   

Gender: 
Posts: 489
|
 |
Re: x^x=5
« Reply #11 on: Jan 24th, 2008, 10:31pm » |
 Quote Quote  Modify Modify
|
Ah yes, ii is multivalued. I must have fallen victim to the same mistake Icarus did. In fact zw is always multivalued unless either z=0 or w=0, even if say z and w are integers, so that we know what zw means in the normal sense.
|
|
 IP Logged IP Logged |
|
|
|
Icarus
wu::riddles Moderator
Uberpuzzler
    

Boldly going where even angels fear to tread.
Gender: 
Posts: 4863
|
 |
Re: x^x=5
« Reply #12 on: Jan 25th, 2008, 6:10pm » |
 Quote Quote  Modify Modify
|
It wasn't the multi-valuedness of the logarithm that was my downfall. I dropped an i from my calculation and so ended up with ei /2 + 2k /2 + 2k i = i for all values of k. i = i for all values of k.
Of course, it was silly not to have noticed such a farcical result even while posting it.
|
|
 IP Logged IP Logged |
"Pi goes on and on and on ...
And e is just as cursed.
I wonder: Which is larger
When their digits are reversed? " - Anonymous
|
|
|
Eigenray
wu::riddles Moderator
Uberpuzzler
    

Gender: 
Posts: 1948
|
 |
Re: x^x=5
« Reply #13 on: Jan 25th, 2008, 8:00pm » |
 Quote Quote  Modify Modify
|
on Jan 24th, 2008, 10:31pm, Obob wrote:| In fact zw is always multivalued unless either z=0 or w=0, even if say z and w are integers, so that we know what zw means in the normal sense. |
|
Even if w is an integer? 
on Jan 25th, 2008, 6:10pm, Icarus wrote:| It wasn't the multi-valuedness of the logarithm that was my downfall. I dropped an i from my calculation and so ended up with ei\subpi/2 + 2k\subpii = i for all values of k. |
|
So what you really showed is that i1 = i, which is a bit more plausible. 
|
|
 IP Logged IP Logged |
|
|
|
Icarus
wu::riddles Moderator
Uberpuzzler
    

Boldly going where even angels fear to tread.
Gender: 
Posts: 4863
|
 |
Re: x^x=5
« Reply #14 on: Jan 25th, 2008, 8:05pm » |
 Quote Quote  Modify Modify
|
Indeed. I showed the full value of my 11 1/2 years of college mathematics to produce this amazing and unexpected result!
|
|
 IP Logged IP Logged |
"Pi goes on and on and on ...
And e is just as cursed.
I wonder: Which is larger
When their digits are reversed? " - Anonymous
|
|
|
Obob
Senior Riddler
   

Gender: 
Posts: 489
|
 |
Re: x^x=5
« Reply #15 on: Jan 25th, 2008, 10:29pm » |
 Quote Quote  Modify Modify
|
Wow, I've been making a lot of mistakes lately. You are of course right Eigenray. For some reason in my back-of-the-envelope calculation I deduced that e2 pi i w = 1 implies w = 0...
|
|
 IP Logged IP Logged |
|
|
|
temporary
Full Member
  

Posts: 255
|
 |
Re: x^x=5
« Reply #16 on: Jan 26th, 2008, 11:08am » |
 Quote Quote  Modify Modify
|
Or any other integer.
|
|
 IP Logged IP Logged |
My goal is to find what my goal is, once I find what my goal is, my goal will be complete.
|
|
|
Icarus
wu::riddles Moderator
Uberpuzzler
    

Boldly going where even angels fear to tread.
Gender: 
Posts: 4863
|
 |
Re: x^x=5
« Reply #17 on: Jan 26th, 2008, 8:10pm » |
 Quote Quote  Modify Modify
|
I think I see the idea behind the ill-formed hint:
If xx = z, then x = z1/x = z1/z^(1/x) = z1/z^(1/(z^(1/x))) = ...
If you make an initial guess x0 for x and form the sequence xn = z^(1/xn-1), and if the sequence converges, it will converge to x.
If the sequence fails to converge, then try xn = ln(z)/ln(xn-1).
The first series converges for both z = 5 and z = i.
For z = i, the value is about 1.36062487029112 + i 1.11943916624235 (using the principle branch of ln(z) when calculating all values).
|
|
 IP Logged IP Logged |
"Pi goes on and on and on ...
And e is just as cursed.
I wonder: Which is larger
When their digits are reversed? " - Anonymous
|
|
|
temporary
Full Member
  

Posts: 255
|
 |
Re: x^x=5
« Reply #18 on: Jan 27th, 2008, 11:25am » |
 Quote Quote  Modify Modify
|
Good, now here is another one. x^x=0. Except this time, I know the answer.
|
|
 IP Logged IP Logged |
My goal is to find what my goal is, once I find what my goal is, my goal will be complete.
|
|
|
Icarus
wu::riddles Moderator
Uberpuzzler
    

Boldly going where even angels fear to tread.
Gender: 
Posts: 4863
|
 |
Re: x^x=5
« Reply #19 on: Jan 27th, 2008, 11:34am » |
 Quote Quote  Modify Modify
|
So does everyone here: there is no solution (not in 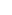 anyway). anyway).
|
| « Last Edit: Jan 27th, 2008, 11:36am by Icarus » |
 IP Logged IP Logged |
"Pi goes on and on and on ...
And e is just as cursed.
I wonder: Which is larger
When their digits are reversed? " - Anonymous
|
|
|
temporary
Full Member
  

Posts: 255
|
 |
Re: x^x=5
« Reply #20 on: Jan 27th, 2008, 3:04pm » |
 Quote Quote  Modify Modify
|
There is a solution. Srn347 might have known it.
|
|
 IP Logged IP Logged |
My goal is to find what my goal is, once I find what my goal is, my goal will be complete.
|
|
|
Obob
Senior Riddler
   

Gender: 
Posts: 489
|
 |
Re: x^x=5
« Reply #21 on: Jan 27th, 2008, 5:49pm » |
 Quote Quote  Modify Modify
|
I've told this to srn347 as well:
Take your crappy nonsense out of Putnam. This is not the place for it.
|
|
 IP Logged IP Logged |
|
|
|
temporary
Full Member
  

Posts: 255
|
 |
Re: x^x=5
« Reply #22 on: Jan 27th, 2008, 7:34pm » |
 Quote Quote  Modify Modify
|
Take your ****** language and attitude out of my thread. This is not a place for it.
|
|
 IP Logged IP Logged |
My goal is to find what my goal is, once I find what my goal is, my goal will be complete.
|
|
|
Icarus
wu::riddles Moderator
Uberpuzzler
    

Boldly going where even angels fear to tread.
Gender: 
Posts: 4863
|
 |
Re: x^x=5
« Reply #23 on: Jan 27th, 2008, 8:53pm » |
 Quote Quote  Modify Modify
|
There is NO solution in 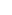 . 0^0 = 1, and if x . 0^0 = 1, and if x 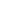 0, then xy 0, then xy 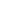 0 either, for any y, and any acceptable definition of exponentiation. 0 either, for any y, and any acceptable definition of exponentiation.
I'm guessing that you are thinking something like (- )- )- . But infinities don't work that way. (- . But infinities don't work that way. (- )- )- is an undefined form, and for very good reason. is an undefined form, and for very good reason.
|
|
 IP Logged IP Logged |
"Pi goes on and on and on ...
And e is just as cursed.
I wonder: Which is larger
When their digits are reversed? " - Anonymous
|
|
|
Icarus
wu::riddles Moderator
Uberpuzzler
    

Boldly going where even angels fear to tread.
Gender: 
Posts: 4863
|
 |
Re: x^x=5
« Reply #24 on: Jan 27th, 2008, 9:10pm » |
 Quote Quote  Modify Modify
|
on Jan 27th, 2008, 5:49pm, Obob wrote:I've told this to srn347 as well:
Take your crappy nonsense out of Putnam. This is not the place for it. |
|
Obob, it really isn't your call what goes here. The x^x = 5 and x^x = i puzzles are entirely appropriate to this forum, even if they are not as abstract as most of the stuff here. There are a number of other problems in this forum that they match quite well with. Admittedly, temporary doesn't understand the issues he brought up, but there is interesting stuff here non-the-less. When I was in college, my text and reference books were mostly written in the 1970s before people started paying attention to the Lambert W function, and since I wasn't involved in this area of study, I never knew anything about it, until I encountered it here.
Temporary's smug misunderstandings can be annoying at times, but he and his idol are not the first to match this description. And there is value here.
|
|
 IP Logged IP Logged |
"Pi goes on and on and on ...
And e is just as cursed.
I wonder: Which is larger
When their digits are reversed? " - Anonymous
|
|
|
|
 WRITE MATH!
WRITE MATH!
 Home
Home  Help
Help  Search
Search  Members
Members  Login
Login  Register
Register WRITE MATH!
WRITE MATH!
 Home
Home  Help
Help  Search
Search  Members
Members  Login
Login  Register
Register