Author |
Topic: Prime Reciprocal Sums (Read 5126 times) |
|
Benoit_Mandelbrot
Junior Member
Almost doesn't count.
Gender: 
Posts: 133
|
 |
Prime Reciprocal Sums
« on: Feb 11th, 2004, 8:36am » |
 Quote  Modify
|
a. Does the sum of the reciprocals of all prime numbers converge on a finite number? (1/2+1/3+1/5+1/7+1/11+...)
b. Does the alternating sum converge on a finite number?
(1/2-1/3+1/5-1/7+1/11-...+...-...)
|
| « Last Edit: Feb 11th, 2004, 8:37am by Benoit_Mandelbrot » |
 IP Logged |
Because of modulo, different bases, and significant digits, all numbers equal each other!
|
|
|
towr
wu::riddles Moderator
Uberpuzzler
Some people are average, some are just mean.
Gender:
Posts: 13730
|
|
Re: Prime Reciprocal Sums
« Reply #1 on: Feb 11th, 2004, 9:59am » |
Quote Modify
|
::based on that the density of primes decreases very fast O( ln(n)/n) I would say both converge..
And I think I also once read somewhere that the first does (which means the second must as well)::
|
|
IP Logged |
Wikipedia, Google, Mathworld, Integer sequence DB
|
|
|
John_Gaughan
Uberpuzzler
Behold, the power of cheese!
    
Gender:
Posts: 767
|
|
Re: Prime Reciprocal Sums
« Reply #2 on: Feb 11th, 2004, 11:32am » |
Quote Modify
|
I don't know for sure, but I think the first one diverges. I remember a calculus professor talking about some of the odd properties of primes once. He was one of those old, absent-minded professors that would go on hour-long tangents about math. Anyway, I cannot prove it either way, but now I'm curious. I'll have to see if I can find a link to back it up. I think it had to do with the infinite sum [sum] 2-x, where x is positive integers. Of course this sum converges on 1, but the one with primes was a little different. ::
edit: changed the infinite sum to what I was really thinking about, not what my caffeine-deprived mind dreamt up!
|
| « Last Edit: Feb 11th, 2004, 6:54pm by John_Gaughan » |
IP Logged |
x = (0x2B | ~0x2B)
x == the_question
|
|
|
John_Gaughan
Uberpuzzler
Behold, the power of cheese!
Gender:
Posts: 767
|
|
Re: Prime Reciprocal Sums
« Reply #3 on: Feb 11th, 2004, 12:39pm » |
Quote Modify
|
I did some calculations, and while they prove nothing, they do suggest that :: there is a convergance for both.
With 1,000 primes:
a. 2.45741
b. 0.269543
With 10,000 primes:
a. 2.70926
b. 0.269602
I wonder if the first one converges on e... ::
|
|
IP Logged |
x = (0x2B | ~0x2B)
x == the_question
|
|
|
towr
wu::riddles Moderator
Uberpuzzler
Some people are average, some are just mean.
Gender:
Posts: 13730
|
|
Re: Prime Reciprocal Sums
« Reply #4 on: Feb 11th, 2004, 1:24pm » |
Quote Modify
|
::from mathworld.wolfram.com/PrimeSums.html: "In 1737, Euler showed that the sum of the reciprocals of the primes diverges"
and further on:
"Despite the divergence of the sum of reciprocal primes, the alternating series (Sloane's A078437) converges (Robinson and Potter 1971, Finch)" [to about 0.2696063619]
Of course it is still open how to prove it.
The first should be the easier to proof, as it is the older by far..::
|
| « Last Edit: Feb 11th, 2004, 1:26pm by towr » |
IP Logged |
Wikipedia, Google, Mathworld, Integer sequence DB
|
|
|
Benoit_Mandelbrot
Junior Member
Almost doesn't count.
Gender:
Posts: 133
|
|
Re: Prime Reciprocal Sums
« Reply #5 on: Feb 11th, 2004, 2:25pm » |
Quote Modify
|
Good work towr.
::One way to prove the divergence of the sum a. is to do it by grouping. You want to group them by their sum is > c, but c will have to be a small constant though. By grouping with 1/8, it should have an infinite groupings. The same can be done with the x-1 that John_Gaughan suggested. Also the integral test will prove the divergence of x-1, as will the Zeta function [zeta](1). The alternating series converges because of the alternating series convergence test. If you have an alternating series of (-1)x+1f(x) or (-1)xf(x), and f(x)->0 as x->[infty] from 1, then the alternating series converges. So (-1)x+1Prime(x) converges.
I also used a range of numbers for c and I came up with a.'s divergence.::
|
|
IP Logged |
Because of modulo, different bases, and significant digits, all numbers equal each other!
|
|
|
Barukh
Uberpuzzler
Gender:
Posts: 2276
|
|
Re: Prime Reciprocal Sums
« Reply #6 on: Feb 12th, 2004, 6:26am » |
Quote Modify
|
Benoit_Mandelbrot, I doubt if grouping may be used to prove the divergence of the first series (because this would probably require too detailed knowledge about primes' distribution).
I am familiar with two different proofs; both use elementary means, but are quite involved. The following page gives a proof, together with "divergence rate".
|
|
IP Logged |
|
|
|
Benoit_Mandelbrot
Junior Member
Almost doesn't count.
Gender:
Posts: 133
|
|
Re: Prime Reciprocal Sums
« Reply #7 on: Feb 12th, 2004, 8:14am » |
Quote Modify
|
I suppose, but I've seen a proof using grouping with c=1. I used 1/8 because it's smaller and because my calculator can't calculate the 300,000 prime number very fast. The alternating series convergence test though is a good proof right? That was the first thing that I proved. It meets all the criteria of a converging alternating sum. The Zeta function can be used quite nicely to determine some convergences though, but not in the way I'd use it for primes by direct comparison.
|
|
IP Logged |
Because of modulo, different bases, and significant digits, all numbers equal each other!
|
|
|
Barukh
Uberpuzzler
Gender:
Posts: 2276
|
|
Re: Prime Reciprocal Sums
« Reply #8 on: Feb 12th, 2004, 9:18am » |
Quote Modify
|
on Feb 12th, 2004, 8:14am, Benoit_Mandelbrot wrote:| I suppose, but I've seen a proof using grouping with c=1. |
|
That's interesting... I would like to see it.
Quote:| The alternating series convergence test though is a good proof right? |
|
Yes, it's perfectly correct. 
|
| « Last Edit: Feb 12th, 2004, 9:20am by Barukh » |
IP Logged |
|
|
|
Benoit_Mandelbrot
Junior Member
Almost doesn't count.
Gender:
Posts: 133
|
|
Re: Prime Reciprocal Sums
« Reply #9 on: Feb 13th, 2004, 6:54am » |
Quote Modify
|
Oops! It's not there, but at mathworld.wolfram.com.
|
| « Last Edit: Feb 19th, 2004, 8:38am by Benoit_Mandelbrot » |
IP Logged |
Because of modulo, different bases, and significant digits, all numbers equal each other!
|
|
|
Benoit_Mandelbrot
Junior Member
Almost doesn't count.
Gender:
Posts: 133
|
|
Re: Prime Reciprocal Sums
« Reply #10 on: Feb 16th, 2004, 11:23am » |
Quote Modify
|
Here is a nice proof that I came up with. We can use the Taylor's Series expansion of arcsin(x)-x when x=1+[epsilon]. They key is that [epsilon][smiley=approx.gif]0, but [epsilon]>0. The inverse sine of any number greater than one will give a non-real number, but in the TS expansion [sqrt](-1) is not involved anywhere. This contradicts the fact that it converges on a finite real number. The nth term of the TS expansion will be less then 1/prime(n), where the first term of the TS is x^3/6. Since x[smiley=approx.gif]1, the first term is 1/6+[epsilon], where the first term of the prime sum is 1/2. The terms of arcsin(x)-x when x=1+[epsilon] decreases faster than 1/prime(x). The sum of the reciprocals of all primes must diverge because of the direct comparison method because the nth term of arcsin(1+[epsilon])-1-[epsilon] of the TS is less then 1/prime(n). The TS can't converge on a finite real number, therefore the sum of all prime number diverges.
|
| « Last Edit: Feb 17th, 2004, 8:35am by Benoit_Mandelbrot » |
IP Logged |
Because of modulo, different bases, and significant digits, all numbers equal each other!
|
|
|
Barukh
Uberpuzzler
Gender:
Posts: 2276
|
on Feb 16th, 2004, 11:23am, Benoit_Mandelbrot wrote:| Here is a nice proof that I came up with. We can use the Taylor's Series expansion of arcsin(x)-x when x=1+[epsilon]... |
|
Unfortunately, your argument doesn’t work (although I liked your approach!)
Here’s why. Look at the attached Taylor series for arcsin(x). In this form, it is an easy excersize to show that for any x > 1 the series contains the minimal term, and therefore is not bounded by the primes' reciprocals from above.
|
|
IP Logged |
|
|
|
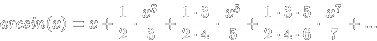
Benoit_Mandelbrot
Junior Member
Almost doesn't count.
Gender:
Posts: 133
|
|
Re: Prime Reciprocal Sums
« Reply #12 on: Feb 19th, 2004, 6:03am » |
Quote Modify
|
In my expansion, I did the Taylor of arcsin(x)-x, not arcsin(x). If arcsin(x) is not purely real but x is, then arcsin(x)-x will still not be purely real. But in the expansion, i isn't present, therefore the Taylor's expansion diverges, and the nth term of arcsin(x)-x when x=1+[epsilon] is less then 1/prime(n). The sum of reciprocals of primes must diverge. Here is the series:
arcsin(x)-x[smiley=approx.gif]
3 5 7 9 11
x 3 x 5 x 35 x 63 x
-- + ---- + ---- + ----- + ------ +...
6 40 112 1152 2816
|
| « Last Edit: Feb 19th, 2004, 8:14am by Benoit_Mandelbrot » |
IP Logged |
Because of modulo, different bases, and significant digits, all numbers equal each other!
|
|
|
Sameer
Uberpuzzler
Pie = pi * e
Gender:
Posts: 1261
|
|
Re: Prime Reciprocal Sums
« Reply #13 on: Feb 19th, 2004, 7:03am » |
Quote Modify
|
Hmm I think I posted something and the site went down!!! 
Here's some easy explanation
Let sigma 1/pk (k goes from 1 to infinity) define our problem and sigma 1/k define the harmonic series.
Obviously sigma 1/pk > sigma 1/k
We know the harmonic series diverges and by virtue of comparison so does the prime series.
|
|
IP Logged |
"Obvious" is the most dangerous word in mathematics.
--Bell, Eric Temple
Proof is an idol before which the mathematician tortures himself.
Sir Arthur Eddington, quoted in Bridges to Infinity
|
|
|
Benoit_Mandelbrot
Junior Member
Almost doesn't count.
Gender:
Posts: 133
|
|
Re: Prime Reciprocal Sums
« Reply #14 on: Feb 19th, 2004, 8:26am » |
Quote Modify
|
There are symbols that can make the viewing easier to understand, but I think you are trying to do
[infty] 1
[sum] ---
k=1 pk , and compare it to
[infty] 1
[sum] ---
k=1 k , am I right? 1/2<1 and 1/3<1/2, and 1/5<1/3, and so on. That method wouldn't work because the nth term of the divergent series must be less then the nth term of the series you want to test. In this case, you can prove the divergence of 1/k, but not the primes.
|
| « Last Edit: Feb 19th, 2004, 8:28am by Benoit_Mandelbrot » |
IP Logged |
Because of modulo, different bases, and significant digits, all numbers equal each other!
|
|
|
Sameer
Uberpuzzler
Pie = pi * e
Gender:
Posts: 1261
|
|
Re: Prime Reciprocal Sums
« Reply #15 on: Feb 19th, 2004, 9:51am » |
Quote Modify
|
sheesh never mind.. i was thinking something else.. it would have worked if it were smaller instead of greater.. 
|
|
IP Logged |
"Obvious" is the most dangerous word in mathematics.
--Bell, Eric Temple
Proof is an idol before which the mathematician tortures himself.
Sir Arthur Eddington, quoted in Bridges to Infinity
|
|
|
Icarus
wu::riddles Moderator
Uberpuzzler
Boldly going where even angels fear to tread.
Gender:
Posts: 4863
|
|
Re: Prime Reciprocal Sums
« Reply #16 on: Feb 19th, 2004, 5:46pm » |
Quote Modify
|
on Feb 19th, 2004, 6:03am, Benoit_Mandelbrot wrote:In my expansion, I did the Taylor of arcsin(x)-x, not arcsin(x). If arcsin(x) is not purely real but x is, then arcsin(x)-x will still not be purely real. But in the expansion, i isn't present, therefore the Taylor's expansion diverges, and the nth term of arcsin(x)-x when x=1+[epsilon] is less then 1/prime(n). The sum of reciprocals of primes must diverge. Here is the series:
arcsin(x)-x[smiley=approx.gif]
3 5 7 9 11
x 3 x 5 x 35 x 63 x
-- + ---- + ---- + ----- + ------ +...
6 40 112 1152 2816
|
|
Taylor Series (or MacLaurin series, since we are expanding about 0) have a radius of convergence R such that for |x| < R, it converges, and for |x|>R it diverges. This Mathworld page gives a nice concise treatment.
For this series (which by the way is =, not [approx], to arcsin(x) - x), the radius of convergence is easily seen to be 1, so yes, it does diverge for x>1. This forms a considerably stronger argument than your "arcsin becomes unreal" one, since all that says is that the series does not represent the arcsin for x>1. It does not necessarily imply that the series does not converge. (The presence of a pole at x=1 also indicates that the series cannot converge for x>1, but that argument requires other tools.)
However, the next part of your argument is far from obvious and needs demonstration: that for some fixed x>1, the terms of the series are less than 1/prime(n).
|
|
IP Logged |
"Pi goes on and on and on ...
And e is just as cursed.
I wonder: Which is larger
When their digits are reversed? " - Anonymous
|
|
|
Barukh
Uberpuzzler
Gender:
Posts: 2276
|
|
Re: Prime Reciprocal Sums
« Reply #17 on: Feb 20th, 2004, 4:04am » |
Quote Modify
|
on Feb 19th, 2004, 5:46pm, Icarus wrote:| However, the next part of your argument is far from obvious and needs demonstration: that for some fixed x>1, the terms of the series are less than 1/prime(n). |
|
That's exactly what I meant: this claim is wrong, and therefore the proof doesn't work.
To be more precise this time: Two Taylor series presented – one in my post, another in Benoit’s post – are the same (except the first element, of course). Just the form of the terms in my expression makes it easy to decide. Let Tn be the n-th term of this series. Then, Tn-1/ Tn = x-22n(2n+1)/(2n-1)2 = x-2[1 + (6n-1)/(2n-1)2], so it’s obvious that for every x > 1 there exists n for which this ratio becomes < 1. Thus, it is not true that Tn [smiley=to.gif] 0, therefore, it is not true that Tn < 1/pn.
|
|
IP Logged |
|
|
|
Icarus
wu::riddles Moderator
Uberpuzzler
Boldly going where even angels fear to tread.
Gender:
Posts: 4863
|
|
Re: Prime Reciprocal Sums
« Reply #18 on: Feb 20th, 2004, 9:06pm » |
Quote Modify
|
And, to reinforce the other point in my previous reply, it is not always true that a Taylor series converges to the function from which it is derived. The classic counter example is the function f(x) = e-1/x^2, for x [ne] 0; f(0) = 0. This function is infinitely differentiable at every point, and it's MacLaurin series has an infinite radius of convergence, but the function it converges to is clearly not f(x). I will leave it to you to actually calculate the series (it's easier than it looks) and discover why.
This example is actually of great use in differential geometry, as it allows you to show the existance of smooth partitions of unity - an extremely useful tool.
|
|
IP Logged |
"Pi goes on and on and on ...
And e is just as cursed.
I wonder: Which is larger
When their digits are reversed? " - Anonymous
|
|
|
Benoit_Mandelbrot
Junior Member
Almost doesn't count.
Gender:
Posts: 133
|
|
Re: Prime Reciprocal Sums
« Reply #19 on: Feb 23rd, 2004, 9:41am » |
Quote Modify
|
That's correct. The series won't converge because x>1, but the function's supposed to converge on a complex number, so the series won't. So the series diverges. The nth term of arcsin(x)-x is less than 1/prime(n). The prime reciprocal sums diverges. The series decreases faster than 1/prime(n) does though, when x[approx]1>1., or 1+[epsilon]. I did about 50 terms with 1+10-10, and it worked. The [vartriangle]x increases.
|
| « Last Edit: Feb 23rd, 2004, 10:04am by Benoit_Mandelbrot » |
IP Logged |
Because of modulo, different bases, and significant digits, all numbers equal each other!
|
|
|
Icarus
wu::riddles Moderator
Uberpuzzler
Boldly going where even angels fear to tread.
Gender:
Posts: 4863
|
|
Re: Prime Reciprocal Sums
« Reply #20 on: Feb 23rd, 2004, 4:15pm » |
Quote Modify
|
No - first of all, the function does not "converge on a complex number". Functions do not converge - limits do. Functions simply have values. For x > 1, the value of arcsin(x) is complex (no matter which branch you take). However, this is not sufficient to prove the series diverges for x>1, not by itself. There is NO a priori guarantee that a Taylor series must converge to its defining function, even when it does converge. In the example I gave, f(x) = e-1/x^2, x [ne] 0; f(0) = 0, the Taylor expansion of f about 0 converges for all x. But the function it converges to is not f. (Try it!)
So, all you have is that arcsin(x) [ne] [sum] anxn for x > 1. This failure to be equal in and of itself does NOT mean that the series diverges.
Now the series does diverge for |x| > 1, but your argument does not prove it.
Quote:| The series decreases faster than 1/prime(n) does though, when x1>1., or 1+. I did about 50 terms with 1+10-10, and it worked. The x increases. |
|
50 terms does not a demonstration make. For this to be a proof, you must show it true for every term. Not just the first 50 or 100 or ... . According to Barukh (whose post I have not checked myself), it isn't true for every term.
|
|
IP Logged |
"Pi goes on and on and on ...
And e is just as cursed.
I wonder: Which is larger
When their digits are reversed? " - Anonymous
|
|
|
Barukh
Uberpuzzler
Gender:
Posts: 2276
|
|
Re: Prime Reciprocal Sums
« Reply #21 on: Feb 25th, 2004, 11:10am » |
Quote Modify
|
Thank you, very much, Icarus! It seems my authority is not enough 
|
|
IP Logged |
|
|
|
Jack Huizenga
Guest
|
|
Re: Prime Reciprocal Sums
« Reply #22 on: Jul 8th, 2004, 4:51pm » |
Quote Modify
 Remove
|
Its really easy to show that the alternating sum of prime reciprocals converges. In general, any sum \sum_{k=1}^\infty a_k where
1. |a_{k+1}|<|a_k| for every k
2. The signs of the a_k are alternating and
3. \lim_{k\to \infty} a_k=0
converges. But setting a_k=(-1)^k/p_k, where p_k denotes the kth prime, we see that a_k is such a sequence and therefore that the sum converges.
|
|
IP Logged |
|
|
|
|
RIDDLES SITE
WRITE MATH!
Home 
Help 
Search 
Members 
Login 
Register
Reply
Notify of replies
Send Topic
Print