 Author
Author |
Topic: sum phi(n)/(2^n - 1) (Read 751 times) |
|
Eigenray
wu::riddles Moderator
Uberpuzzler
    

Gender: 
Posts: 1948
|
 |
sum phi(n)/(2^n - 1)
« on: Sep 9th, 2009, 9:23pm » |
 Quote Quote  Modify Modify
|
Evaluate:
 n=1 n=1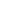  (n)/(2n-1) (n)/(2n-1)
|
|
 IP Logged IP Logged |
|
|
|
william wu
wu::riddles Administrator
    


Gender: 
Posts: 1291
|
 |
Re: sum phi(n)/(2^n - 1)
« Reply #1 on: Sep 13th, 2009, 2:43pm » |
 Quote Quote  Modify Modify
|
Here is an upper bound that matches numerical calculation of the limit (thanks Eigenray):
| hidden: |
We first prove the following inequality: n/2n 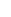 phi(n)/(2^n - 1) for n > 1. phi(n)/(2^n - 1) for n > 1.
By rearranging, the inequality we wish to show can be rewritten as 1 - (1/2)^n 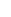 phi(n)/n. phi(n)/n.
This holds since
phi(n)/n
=  p|n (1-1/p) p|n (1-1/p)
<= 1-1/n
<= 1 - (1/2)^n
where the first inequality holds since n>1, and the second inequality holds since n <= 2^n.
Thus, applying this inequality to every term of our series except the first term, we have
 n=1 to n=1 to  phi(n) / (2^n - 1) phi(n) / (2^n - 1)
<= 1 +  n=2 to n=2 to  n/2n n/2n
= 1 + -1/2 +  n=1 to n=1 to  n/2n n/2n
= 2.5
In the last equality, the sum  n/2^n can be evaluated by using the trick of differentiating the geometric series n/2^n can be evaluated by using the trick of differentiating the geometric series  x^n = 1/(1-x) when |x|<1. In this way, we can show that x^n = 1/(1-x) when |x|<1. In this way, we can show that  n=1 to n=1 to  n x^n = x/(1-x)^2, which evaluates to 2 when x = 1/2. n x^n = x/(1-x)^2, which evaluates to 2 when x = 1/2.
|
|
| « Last Edit: Sep 18th, 2009, 5:43am by william wu » |
 IP Logged IP Logged |
[ wu ] : http://wuriddles.com / http://forums.wuriddles.com
|
|
|
Eigenray
wu::riddles Moderator
Uberpuzzler
    

Gender: 
Posts: 1948
|
 |
Re: sum phi(n)/(2^n - 1)
« Reply #2 on: Sep 13th, 2009, 5:41pm » |
 Quote Quote  Modify Modify
|
The only way the upper bound could be sharp is if it were sharp term by term, which it isn't. The upper bound you give is actually
1 - 1/2 + 2 = 2.5
Hint: I intentionally made the problem more difficult by making it 'easier', i.e., asking for less information.
|
|
 IP Logged IP Logged |
|
|
|
Eigenray
wu::riddles Moderator
Uberpuzzler
    

Gender: 
Posts: 1948
|
 |
Re: sum phi(n)/(2^n - 1)
« Reply #3 on: Sep 21st, 2009, 2:04am » |
 Quote Quote  Modify Modify
|
Here is the 'harder' (easier) version: if x > 1, evaluate:
  (n)/(xn-1) (n)/(xn-1)
|
|
 IP Logged IP Logged |
|
|
|
|
 WRITE MATH!
WRITE MATH!
 Home
Home  Help
Help  Search
Search  Members
Members  Login
Login  Register
Register WRITE MATH!
WRITE MATH!
 Home
Home  Help
Help  Search
Search  Members
Members  Login
Login  Register
Register