 Author
Author |
Topic: Two Intersecting Spheres (Read 506 times) |
|
ThudnBlunder
wu::riddles Moderator
Uberpuzzler
    

The dewdrop slides into the shining Sea
Gender: 
Posts: 4489
|
 |
Two Intersecting Spheres
« on: Oct 21st, 2008, 3:00pm » |
 Quote Quote  Modify Modify
|
S and T, with radii r and R respectively, are two intersecting spheres such that S passes through the centre of T. If R 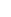 2r find the area of the surface of S that lies inside T. 2r find the area of the surface of S that lies inside T.
|
|
 IP Logged IP Logged |
THE MEEK SHALL INHERIT THE EARTH.....................................................................er, if that's all right with the rest of you.
|
|
|
Grimbal
wu::riddles Moderator
Uberpuzzler
    

I am sorry Dave, I *can* do that.
Gender: 
Posts: 7528
|
 |
Re: Two Intersecting Spheres
« Reply #1 on: Oct 21st, 2008, 3:48pm » |
 Quote Quote  Modify Modify
|
Surprising result, looks wrong at first, and yet it works.
|
|
 IP Logged IP Logged |
|
|
|
towr
wu::riddles Moderator
Uberpuzzler
    

Some people are average, some are just mean.
Gender: 
Posts: 13730
|
 |
Re: Two Intersecting Spheres
« Reply #2 on: Oct 22nd, 2008, 1:45am » |
 Quote Quote  Modify Modify
|
Guessing, pi R2?
Works at 3 points, certainly.
R=0; quite obvious.
R=sqrt(2) r: 2 pi r2, half the sphere S.
R=2 r: 4 pi r2, whole sphere S.
|
| « Last Edit: Oct 22nd, 2008, 1:46am by towr » |
 IP Logged IP Logged |
Wikipedia, Google, Mathworld, Integer sequence DB
|
|
|
SMQ
wu::riddles Moderator
Uberpuzzler
    

Gender: 
Posts: 2084
|
 |
Re: Two Intersecting Spheres
« Reply #3 on: Oct 22nd, 2008, 6:21am » |
 Quote Quote  Modify Modify
|
Good guess, towr!
Let point O be the center of sphere S and point P be the center of sphere T. Take any plane passing through both O and P, and let point Q be one of the two points where S and T intersect in the plane. Triangle OPQ has sides of r, r and R, and so angle POQ has value 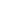 = 2sin-1(R/2r). = 2sin-1(R/2r).
The surface area of S within T is then  0 0 2 2 r sin( r sin( ) r d ) r d
= 2 r2 r2  0 0 sin( sin( ) d ) d
= 2 r2(1 - cos(2sin-1(R/2r))) r2(1 - cos(2sin-1(R/2r)))
= 2 r2(1 - (1 - 2sin2(sin-1(R/2r)))) r2(1 - (1 - 2sin2(sin-1(R/2r))))
= 4 r2(R/2r)2 r2(R/2r)2
=  R2 R2
--SMQ
|
|
 IP Logged IP Logged |
--SMQ
|
|
|
|
 WRITE MATH!
WRITE MATH!
 Home
Home  Help
Help  Search
Search  Members
Members  Login
Login  Register
Register WRITE MATH!
WRITE MATH!
 Home
Home  Help
Help  Search
Search  Members
Members  Login
Login  Register
Register