 Author
Author |
Topic: Functional equation (Read 608 times) |
|
wonderful
Full Member
  

Posts: 203
|
 |
Functional equation
« on: Sep 2nd, 2008, 1:32pm » |
 Quote Quote  Modify Modify
|
Can you find f(x): R--> R such that
f(x+f(y)) = f(y+f(x)) for every x, y in R?
Have A Great Day!
|
|
 IP Logged IP Logged |
|
|
|
pex
Uberpuzzler
    

Gender: 
Posts: 880
|
 |
Re: Functional equation
« Reply #2 on: Sep 2nd, 2008, 2:18pm » |
 Quote Quote  Modify Modify
|
on Sep 2nd, 2008, 2:12pm, towr wrote:Very easily? f(x)=x
And of course f(x)=0 |
|
And both of these plus an arbitrary real constant.
At least, if there are other solutions, they cannot be injective:
| hidden: | Let f(x) be an injective solution.
Thus, f(x+f(y)) = f(y+f(x)) implies x+f(y) = y+f(x).
But then, for all x,y, f(x)-f(y) = x-y.
Or, for all x and y not equal, [f(x)-f(y)]/[x-y] = 1.
Taking the limit as y -> x, f'(x) exists and is equal to 1 for all x.
Thus, f(x) = x + constant. |
|
|
 IP Logged IP Logged |
|
|
|
wonderful
Full Member
  

Posts: 203
|
 |
Re: Functional equation
« Reply #3 on: Sep 2nd, 2008, 2:42pm » |
 Quote Quote  Modify Modify
|
Great towr and pex! I also really like Pex's solution.
Have A Great Day!
|
|
 IP Logged IP Logged |
|
|
|
pex
Uberpuzzler
    

Gender: 
Posts: 880
|
 |
Re: Functional equation
« Reply #4 on: Sep 2nd, 2008, 2:54pm » |
 Quote Quote  Modify Modify
|
on Sep 2nd, 2008, 2:42pm, wonderful wrote:Great towr and pex! I also really like Pex's solution.
Have A Great Day! |
|
 I wouldn't consider this one solved yet... I wouldn't consider this one solved yet...
|
|
 IP Logged IP Logged |
|
|
|
SMQ
wu::riddles Moderator
Uberpuzzler
    

Gender: 
Posts: 2084
|
 |
Re: Functional equation
« Reply #5 on: Sep 2nd, 2008, 3:43pm » |
 Quote Quote  Modify Modify
|
Sure, pex proved the only injective solution is f(x) = x + C, but there are all kinds of non-injective solutions left to find!
towr notes f(x) = C as a trivial non-injective example. I'll also note f(x) =  x x + D, D + D, D   and related families are solutions, as are f(x) = (x + C) mod m, m and related families are solutions, as are f(x) = (x + C) mod m, m  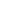 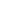 0 and related families. I'm sure there are many more as well -- pretty much any function that maps 0 and related families. I'm sure there are many more as well -- pretty much any function that maps 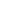  X X 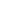 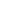 in a "periodic", "non-stretchy" way should work! in a "periodic", "non-stretchy" way should work!
--SMQ
|
|
 IP Logged IP Logged |
--SMQ
|
|
|
Obob
Senior Riddler
   

Gender: 
Posts: 489
|
 |
Re: Functional equation
« Reply #6 on: Sep 3rd, 2008, 3:21pm » |
 Quote Quote  Modify Modify
|
Consider the following function:
f(x)=x if x is irrational
f(x)=0 if x is rational.
Then
f(x+f(y))=0 if x is rational and y is rational
f(x+f(y))=x if x is irrational and y is rational
f(x+f(y))=y if x is rational and y is irrational
f(x+f(y))=x+y if x is irrational, y is irrational, and x+y is irrational
f(x+f(y))=0 if x is irrational, y is irrational, and x+y is rational
from which it follows that f(x+f(y))=f(y+f(x)) in all cases. This gives a solution which is continuous at exactly 1 point.
This example can also be "composed" with SMQ's periodic examples, for instance by defining f(x) to be the remainder r of x with 0<= r < m after dividing by a rational (maybe irrational too, haven't thought enough about it) real number m whenever x is irrational and 0 otherwise.
More generally, if S is any subgroup of the real numbers under addition, it can take the place of the rationals in my construction.
For a much nastier example, choose a set of coset representatives for R/Q (or more generally R/S). Every element of R belongs to a unique coset of R/Q, so we can define a function R->R assigning a real number to the coset representative of the coset containing it. This function isn't even measurable.
Conceptually we can think of this construction as follows. If h:R->A is a homomorphism of abelian groups and A admits a function g satisfying the functional equation g(x+g(y))=g(y+g(x)) for all x,y, then any function s:A->R satisfying hs= id_A (i.e. any section, which we only require to be a function and not a homomorphism) induces a function f:R->R satisfying the functional equation. Specifically, f is given by f=sgh. Indeed, then
f(x+f(y))=sgh(x+sgh(y))=sg(h(x)+hsgh(y))=sg(h(x)+gh(y))=sg(h(y)+gh(x))=f (y+f(x)).
In our last example, h is the quotient map R->R/Q, g is the identity R/Q->R/Q, and s corresponds to the selection of a choice of coset representatives, where we have chosen the representative of Q to be 0.
For more down-to-earth examples, we could look at the homomorphism R->R/Z, with section given by the inclusion of R/Z into R as numbers x satisfying 0<=x<1. Again the identity R/Z->R/Z satisfies the functional equation, and the corresponding f:R->R is given by x->x (mod 1).
How much worse can these constructions get? Is f(x)=x+C the only nonconstant continuous solution?
It does follow from pex's argument that if f is continuously differentiable in a neighborhood of a point, then the derivative must be either 0 or 1. For if the derivative is nonzero, then f is locally injective and pex's argument applies to show that in fact the derivative is 1. Hence the only nonconstant globally continuously differentiable function f satisfying the equation is f(x)=x+C.
|
| « Last Edit: Sep 3rd, 2008, 5:06pm by Obob » |
 IP Logged IP Logged |
|
|
|
Grimbal
wu::riddles Moderator
Uberpuzzler
    

I am sorry Dave, I *can* do that.
Gender: 
Posts: 7528
|
 |
Re: Functional equation
« Reply #7 on: Sep 4th, 2008, 1:00am » |
 Quote Quote  Modify Modify
|
on Sep 3rd, 2008, 3:21pm, Obob wrote:Consider the following function:
f(x)=x if x is irrational
f(x)=0 if x is rational.
Then
...
f(x+f(y))=y if x is rational and y is irrational
|
|
Hm...
if x is rational and y is irrational
f(x+f(y)) = f(x+y) = x+y
but
f(y+f(x)) = f(y+0) = y
so we don't have
f(x+f(y)) = f(y+f(x))
|
|
 IP Logged IP Logged |
|
|
|
Obob
Senior Riddler
   

Gender: 
Posts: 489
|
 |
Re: Functional equation
« Reply #8 on: Sep 4th, 2008, 1:22am » |
 Quote Quote  Modify Modify
|
My bad. You're right Grimbal.
The pathological example that I give later does work, however. In particular, the one where you pick coset representatives of R/Q.
|
|
 IP Logged IP Logged |
|
|
|
|
 WRITE MATH!
WRITE MATH!
 Home
Home  Help
Help  Search
Search  Members
Members  Login
Login  Register
Register WRITE MATH!
WRITE MATH!
 Home
Home  Help
Help  Search
Search  Members
Members  Login
Login  Register
Register