 Author
Author |
Topic: find x (Read 589 times) |
|
tony123
Junior Member
 

Posts: 61
|
x is acute angle and
sin 5x sin 4x=sin 3x sin 8x
find x
|
|
 IP Logged IP Logged |
|
|
|
Barukh
Uberpuzzler
    

Gender: 
Posts: 2276
|
 |
Re: find x
« Reply #1 on: Mar 3rd, 2008, 1:58am » |
 Quote Quote  Modify Modify
|
45?
... and also 10,50.
|
| « Last Edit: Mar 3rd, 2008, 3:02am by Barukh » |
 IP Logged IP Logged |
|
|
|
Hippo
Uberpuzzler
    

Gender: 
Posts: 919
|
 |
Re: find x
« Reply #2 on: Mar 8th, 2008, 3:34am » |
 Quote Quote  Modify Modify
|
Of course making sin 4x=sin 8x=0 is the easiest. (pi/4) the other solutions are pi/18, 5pi/18 and 7pi/18, but I obtained them by observing computed graph ... So pi/36*(2,9,10,14) ... 10,45,50,70 degrees
Is there a systematic approach? ... Polynomial in sin x?
|
|
 IP Logged IP Logged |
|
|
|
Aryabhatta
Uberpuzzler
    

Gender: 
Posts: 1321
|
 |
Re: find x
« Reply #3 on: Mar 8th, 2008, 4:51am » |
 Quote Quote  Modify Modify
|
Not sure if there is a systematic approach, but the following works for given equation:
if sin 4x = 0, then the equality is satisfied. (x = 45 degrees)
Assume sin 4x is non zero.
Then we have
sin5x sin4x = sin3x * 2* sin4x * cos4x
Thus sin 5x = 2 * sin 3x * cos 4x
Now 2 sin A cos B = sin (A+B) + sin (A-B)
So
sin 5x = sin 7x - sin x
Thus sin x = sin 7x - sin 5x
Now sinA - sin B = 2 cos (A/2+B/2) sin (A/2 - B/2)
Thus
sin x = 2 cos 6x sin x
Since sin x is non-zero for acute x, we get
cos 6x = 1/2 whose solutions are 10, 50, 70 degrees.
All we now have to do is check is if they satisfy the original...(in fact we don't have to, as we can justify that we have been making equivalent transformations)
|
|
 IP Logged IP Logged |
|
|
|
Hippo
Uberpuzzler
    

Gender: 
Posts: 919
|
 |
Re: find x
« Reply #4 on: Mar 8th, 2008, 6:53am » |
 Quote Quote  Modify Modify
|
 Well done ... I have reduced sin 4x case and got to find root of polynomial in odd powers of sin x and cos ^2 x ... in odd powers of sin x. As sin x <> 0 I divided it by sin x and got a polynomial in sin ^2 x. (Actually in (2 sin x)^2.) Well done ... I have reduced sin 4x case and got to find root of polynomial in odd powers of sin x and cos ^2 x ... in odd powers of sin x. As sin x <> 0 I divided it by sin x and got a polynomial in sin ^2 x. (Actually in (2 sin x)^2.)
This was cubic polynomial ... your solution was better.
|
|
 IP Logged IP Logged |
|
|
|
Eigenray
wu::riddles Moderator
Uberpuzzler
    

Gender: 
Posts: 1948
|
 |
Re: find x
« Reply #5 on: Mar 8th, 2008, 10:23am » |
 Quote Quote  Modify Modify
|
Another approach, which requires a bit less ingenuity: write z = eix. Then zn = cos(nx) + i sin(nx), so
cos(nx) = Re[zn] = (zn + 1/zn)/2,
sin(nx) = Im[zn] = (zn - 1/zn)/2i.
From sin(5x) = 2 sin(3x) cos(4x), we get
(z5 - 1/z5)/(2i) = 2 (z3-1/z3)/(2i) (z4+1/z4)/2,
or multiplying by 2i*z7,
z12 - z2 = (z6 - 1)(z8 + 1).
We know that both sides are 0 when x=0, , which correspond to z= , which correspond to z= 1, so dividing both sides by z2-1, we get 1, so dividing both sides by z2-1, we get
z2(z8+z6+z4+z2+1) = (z4+z2+1)(z8+1),
or
z12 - z6 + 1 = 0,
i.e., z6 = (1 i i 3)/2 = e 3)/2 = e 2 2 i/6, and x = ( i/6, and x = ( 2 2 i/6 + 2 i/6 + 2 k)/6. Or, z6 is a primitive 6-th root of unity, so z is a primitive 36-th root of unity: x = 2 k)/6. Or, z6 is a primitive 6-th root of unity, so z is a primitive 36-th root of unity: x = 2 k/36, where k is relatively prime to 36. These are the solutions with sin(4x) k/36, where k is relatively prime to 36. These are the solutions with sin(4x) 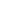 0. 0.
The original equation sin5x sin4x - sin3x sin8x = 0 is equivalent to
(z5-1/z5)(z4-1/z4) - (z3-1/z3)(z8-1/z8)
being 0, which factors as
(z-1)2(z+1)2(z2+1)(z4+1) (z12-z6+1)
= (z2-1)(z8-1)(z12-z6+1) =
= [ 1 1 2][ 2][ 1 1 2 2 4 4 8] 8] 36 36
where  n is the n-th cyclotomic polynomial. This means that z is either a 1st, 2nd, 4th, 8th, or 36th root of unity, where the 1st and 2nd roots of unity (z= n is the n-th cyclotomic polynomial. This means that z is either a 1st, 2nd, 4th, 8th, or 36th root of unity, where the 1st and 2nd roots of unity (z= 1, i.e., sin x=0) have multiplicity 2 (since (sin x)2 divides both sides). Note that the roots of (z2-1) correspond to sinx=0, and the roots of (z8-1) (which include those of z2-1) correspond to sin4x=0. 1, i.e., sin x=0) have multiplicity 2 (since (sin x)2 divides both sides). Note that the roots of (z2-1) correspond to sinx=0, and the roots of (z8-1) (which include those of z2-1) correspond to sin4x=0.
|
|
 IP Logged IP Logged |
|
|
|
|
 WRITE MATH!
WRITE MATH!
 Home
Home  Help
Help  Search
Search  Members
Members  Login
Login  Register
Register WRITE MATH!
WRITE MATH!
 Home
Home  Help
Help  Search
Search  Members
Members  Login
Login  Register
Register