 Author
Author |
Topic: Divide Two Sets Of Quadratic, Get Integers (Read 262 times) |
|
K Sengupta
Senior Riddler
   

Gender: 
Posts: 371
|
 |
Divide Two Sets Of Quadratic, Get Integers
« on: Jul 24th, 2007, 8:01am » |
 Quote Quote  Modify Modify
|
Analytically determine all possible pairs of positive integers (a, b) such that :
(a + b2)/(a2-b) and (a2+b)/(b2-a) are both positive integers.
|
| « Last Edit: Jul 24th, 2007, 8:17am by K Sengupta » |
 IP Logged IP Logged |
|
|
|
Eigenray
wu::riddles Moderator
Uberpuzzler
    

Gender: 
Posts: 1948
|
 |
Re: Divide Two Sets Of Quadratic, Get Intege
« Reply #1 on: Jul 26th, 2007, 2:41pm » |
 Quote Quote  Modify Modify
|
| hidden: | If a=b, then (a+a2)/(a2-a) = (a+1)/(a-1) = 1 + 2/(a-1), so a-1 | 2, so we have a=b=2 or a=b=3.
Otherwise, WLOG suppose a<b.
(a2+b)/(b2-a) 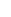 1, so if a 1, so if a  (b-2), then we would have (b-2), then we would have
b2-(b-2)  b2-a b2-a  a2+b a2+b  (b-2)2+b, (b-2)2+b,
or 2b  2, giving b=1 and a<0, a contradiction. 2, giving b=1 and a<0, a contradiction.
So we must have a=b-1. So (b2+b-1)/(b2-3b+1) is an integer. Since it's clearly > 1, it must be at least 2, so
b2+b-1 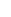 2(b2-3b+1), or 2(b2-3b+1), or
b2-7b+3  0, 0,
which gives b < 7. Checking b=3,4,5,6, the only solution is (a,b) = (2,3). |
|
|
 IP Logged IP Logged |
|
|
|
|
 WRITE MATH!
WRITE MATH!
 Home
Home  Help
Help  Search
Search  Members
Members  Login
Login  Register
Register WRITE MATH!
WRITE MATH!
 Home
Home  Help
Help  Search
Search  Members
Members  Login
Login  Register
Register