 Author
Author |
Topic: Consider The Minimum, Get 98 Zeroes (Read 279 times) |
|
K Sengupta
Senior Riddler
   

Gender: 
Posts: 371
|
 |
Consider The Minimum, Get 98 Zeroes
« on: Jul 24th, 2007, 7:19am » |
 Quote Quote  Modify Modify
|
Analytically determine the positive integers a and b , so that ab is minimum with the proviso that the last 98 digits in the decimal expansion of aa*bb are all zeroes.
|
|
 IP Logged IP Logged |
|
|
|
SMQ
wu::riddles Moderator
Uberpuzzler
    

Gender: 
Posts: 2084
|
 |
Re: Consider The Minimum, Get 98 Zeroes
« Reply #1 on: Jul 24th, 2007, 1:47pm » |
 Quote Quote  Modify Modify
|
At least the last 98, or only the last 98? In the former case, I don't see a way to beat the trivial solution of 1, 100; in the latter case, the family 75+150n, 98 (where n = 0 gives the obvious minimum) seem to be the only solutions.
--SMQ
|
|
 IP Logged IP Logged |
--SMQ
|
|
|
Eigenray
wu::riddles Moderator
Uberpuzzler
    

Gender: 
Posts: 1948
|
 |
Re: Consider The Minimum, Get 98 Zeroes
« Reply #2 on: Jul 24th, 2007, 2:58pm » |
 Quote Quote  Modify Modify
|
SMQ, I get the same minimum, but your general solution only gives ~ 1/15 of the solutions.
| hidden: |
The number of times 5 divides n=aabb is v5(n) = a*v5(a)+b*v5(b), which is evidently divisible by 5. Since v5(n) 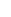 98, in fact v5(n) 98, in fact v5(n) 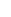 100. If only the last 98 digits of n are 0, then we must therefore have v2(n) = 98. Therefore only one of a,b can be divisible by 5; WLOG say 5|a. 100. If only the last 98 digits of n are 0, then we must therefore have v2(n) = 98. Therefore only one of a,b can be divisible by 5; WLOG say 5|a.
If a is even, then since v2(n)=98, we must have a < 100. But a*v5(a) 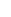 100, so a must be divisible by 25. The only possibility then is a=50, and so we need b*v2(b) = 48. But if b=2rm, with m odd, this gives r*2rm=48=24*3. So we need r + v2(r) = 4, and checking r=1,2,3,4 shows this is impossible. This contradiction shows that a is odd. 100, so a must be divisible by 25. The only possibility then is a=50, and so we need b*v2(b) = 48. But if b=2rm, with m odd, this gives r*2rm=48=24*3. So we need r + v2(r) = 4, and checking r=1,2,3,4 shows this is impossible. This contradiction shows that a is odd.
We therefore have b*v2(b) = 98; setting b=2rm as before gives r+v2(r)=1, so r=1, and b=98.
Now a*v5(a) 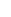 100. If a < 100, then 25|a, and the only possibility is a=75. For a > 100, any odd number divisible by 5 will do. So the possibilities for a are: 75, or {105+10k, k 100. If a < 100, then 25|a, and the only possibility is a=75. For a > 100, any odd number divisible by 5 will do. So the possibilities for a are: 75, or {105+10k, k 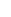 0}. 0}. |
|
|
 IP Logged IP Logged |
|
|
|
SMQ
wu::riddles Moderator
Uberpuzzler
    

Gender: 
Posts: 2084
|
 |
Re: Consider The Minimum, Get 98 Zeroes
« Reply #3 on: Jul 24th, 2007, 4:22pm » |
 Quote Quote  Modify Modify
|
Yeah, I realized after I posted it and before I could get back to a computer that 98, any odd multiple of 5 > 98 would work.
--SMQ
|
| « Last Edit: Jul 24th, 2007, 4:22pm by SMQ » |
 IP Logged IP Logged |
--SMQ
|
|
|
|
 WRITE MATH!
WRITE MATH!
 Home
Home  Help
Help  Search
Search  Members
Members  Login
Login  Register
Register WRITE MATH!
WRITE MATH!
 Home
Home  Help
Help  Search
Search  Members
Members  Login
Login  Register
Register