Author |
Topic: Train Rod (Read 1750 times) |
|
ThudnBlunder
wu::riddles Moderator
Uberpuzzler
The dewdrop slides into the shining Sea
Gender: 
Posts: 4489
|
Suppose a train travels between two railway stations along a straight track. A straight rigid rod is hinged to the floor of one of the carriages, able to move without friction either forward or backward until it touches the floor. If it does touch the floor, assume it remains there. Suppose it is specified in advance how the train will move. The motion need not be uniform; it can speed up, stop suddenly, or even reverse for a while. But it must start at one station and end at the other. Can you always place the rod in such a position that it never hits the floor during its journey?
Well, the final position of the rod depends continuously on its initial position. And there's a continuous range of angles that it can start at, from 0o to 180o; hence the range of final angles is also continuous. If it starts lying down forwards at 0o it stays there and if it starts lying down backwards at 180o it stays there. So the range of final angles includes all values between 0o and 180o. In particular, it includes 90o; so it can be arranged for the rod to finish up vertically. Since it remains on the floor when it hits it, it won't hit the floor at all.
Right or wrong?
|
| « Last Edit: May 22nd, 2007, 11:47am by ThudnBlunder » |
 IP Logged |
THE MEEK SHALL INHERIT THE EARTH.....................................................................er, if that's all right with the rest of you.
|
|
|
Icarus
wu::riddles Moderator
Uberpuzzler
Boldly going where even angels fear to tread.
Gender:
Posts: 4863
|
 |
Re: Train Rod
« Reply #1 on: May 13th, 2007, 7:39pm » |
 Quote  Modify
|
on May 13th, 2007, 6:53pm, ThudanBlunder wrote:| Well, the final position of the rod depends continuously on its initial position. |
|
Says who?
|
|
IP Logged |
"Pi goes on and on and on ...
And e is just as cursed.
I wonder: Which is larger
When their digits are reversed? " - Anonymous
|
|
|
ThudnBlunder
wu::riddles Moderator
Uberpuzzler
The dewdrop slides into the shining Sea
Gender:
Posts: 4489
|
|
Re: Train Rod
« Reply #2 on: May 13th, 2007, 8:11pm » |
Quote Modify
|
on May 13th, 2007, 7:39pm, Icarus wrote:
The accused are Richard Courant and Herbert Robbins.
|
|
IP Logged |
THE MEEK SHALL INHERIT THE EARTH.....................................................................er, if that's all right with the rest of you.
|
|
|
Icarus
wu::riddles Moderator
Uberpuzzler
Boldly going where even angels fear to tread.
Gender:
Posts: 4863
|
|
Re: Train Rod
« Reply #3 on: May 13th, 2007, 8:11pm » |
Quote Modify
|
Actually, I take that back. My thought was that if you take the same rod situation and attach it to a wall, any initial position except straight up will result in the rod pointing straight down. If the rod starts out straight up, it stays that way. So in this case, the final position of the rod does not vary continuously with the initial position. However, this works because the ratio of the force pulling the rod down is infinitely larger than that pulling it towards the wall, as the latter is zero.
If we put the lever back on the floor again, this is no longer the case. The force pulling the lever to the floor is constant and positive, so the force along the train is no longer infinitely stronger. And because the behavior of the train is fixed (something I had overlooked), there is a maximum to this ratio, which ultimately means the variance is continuous.
|
|
IP Logged |
"Pi goes on and on and on ...
And e is just as cursed.
I wonder: Which is larger
When their digits are reversed? " - Anonymous
|
|
|
Barukh
Uberpuzzler
Gender:
Posts: 2276
|
|
Re: Train Rod
« Reply #4 on: May 13th, 2007, 11:09pm » |
Quote Modify
|
Right
|
|
IP Logged |
|
|
|
towr
wu::riddles Moderator
Uberpuzzler
Some people are average, some are just mean.
Gender:
Posts: 13730
|
|
Re: Train Rod
« Reply #5 on: May 14th, 2007, 1:13am » |
Quote Modify
|
I can see a number of practical problems. And chaos would throw off any attempt at it.
Also, I'd expect the rod to bounce on the floor if it fell on it. So I wouldn't expect it to stay at 0 or 180 degrees if it went there.
I suppose it's a mathematical rod though, which changes things a little.
|
|
IP Logged |
Wikipedia, Google, Mathworld, Integer sequence DB
|
|
|
Grimbal
wu::riddles Moderator
Uberpuzzler
Gender:
Posts: 7527
|
|
Re: Train Rod
« Reply #6 on: May 14th, 2007, 1:56am » |
Quote Modify
|
You have to take into account that butterfly in Beijing.
|
|
IP Logged |
|
|
|
ThudnBlunder
wu::riddles Moderator
Uberpuzzler
The dewdrop slides into the shining Sea
Gender:
Posts: 4489
|
|
Re: Train Rod
« Reply #7 on: May 14th, 2007, 6:46am » |
Quote Modify
|
on May 14th, 2007, 1:56am, Grimbal wrote:| You have to take into account that butterfly in Beijing. |
|
Yes, this puzzle is about a c-word, but it ain't 'chaos', quite the opposite. Nuff said.
|
| « Last Edit: May 14th, 2007, 7:23am by ThudnBlunder » |
IP Logged |
THE MEEK SHALL INHERIT THE EARTH.....................................................................er, if that's all right with the rest of you.
|
|
|
rmsgrey
Uberpuzzler
    
Gender:
Posts: 2873
|
|
Re: Train Rod
« Reply #8 on: May 14th, 2007, 8:17am » |
Quote Modify
|
The rod's final position depends continuously on its initial position iff it's possible to position it so that it never touches the floor.
An important question is whether the train is required to arrive in a finite time - if it is, since the rod's new position depends continuously on its old position over short finite intervals, the final position over the run will also depend continuously. If the train is allowed to take a non-finite time (infinite or infinitesimal) to arrive, then the rod's final position will not (in general) depend continuously on the initial position.
|
|
IP Logged |
|
|
|
Eigenray
wu::riddles Moderator
Uberpuzzler
Gender:
Posts: 1948
|
|
Re: Train Rod
« Reply #9 on: May 15th, 2007, 12:12am » |
Quote Modify
|
on May 14th, 2007, 8:17am, rmsgrey wrote:| An important question is whether the train is required to arrive in a finite time |
|
Indeed, when I first read it I thought: how could it be continuous when there are only 3 possible outcomes? But then I realized that the time it takes the rod to fall when offset slightly from vertical is in fact unbounded.
|
|
IP Logged |
|
|
|
ThudnBlunder
wu::riddles Moderator
Uberpuzzler
The dewdrop slides into the shining Sea
Gender:
Posts: 4489
|
|
Re: Train Rod
« Reply #10 on: May 18th, 2007, 11:48am » |
Quote Modify
|
on May 14th, 2007, 8:17am, rmsgrey wrote:| An important question is whether the train is required to arrive in a finite time |
|
It is.
But consider all possible histories if there were no absorbing boundary conditions That is, that the rod could turn a full 360o (no floor). When they are replaced any initial position ends up on the floor at either 0o or 360o. Thus the assumption of continuity is wrong and the answer is 'wrong'.
See "Au Courant with Differential Equations", Manifold #18 (Spring 1976), pp. 6-9 by Tim Posten.
|
| « Last Edit: May 18th, 2007, 11:53am by ThudnBlunder » |
IP Logged |
THE MEEK SHALL INHERIT THE EARTH.....................................................................er, if that's all right with the rest of you.
|
|
|
Eigenray
wu::riddles Moderator
Uberpuzzler
Gender:
Posts: 1948
|
|
Re: Train Rod
« Reply #11 on: May 18th, 2007, 2:21pm » |
Quote Modify
|
on May 18th, 2007, 11:48am, ThudanBlunder wrote:| When they are replaced any initial position ends up on the floor at either 0o or 360o. Thus the assumption of continuity is wrong and the answer is 'wrong'. |
|
I don't follow this.
Leonard Gillman reviewed What Is Mathematics? in The American Mathematical Monthly, Vol. 105, No. 5 (May, 1998), pp. 485-488). Here's what he has to say about this problem, which he attributes to Hassler Whitney (via Acrobat Reader's text extraction, with limited editting):
Then there is the matter of the freight-car problem (topic 9). Here is the original presentation:
Suppose a train travels from station A to station B along a straight section of track. The journey need not be of uniform speed or acceleration. The train may act in any manner, speeding up, slowing down, coming to a halt, or even backing up for a while, before reaching B. But the exact motion of the train is supposed to be known in advance; that is, the function s = f(t) is given, where s is the distance of the train from station A , and t is the time, measured from the instant of departure. On the floor of one of the cars a rod is pivoted so that it may move without friction either forward or backward until it touches the floor. If it does touch the floor, we assume that it remains on the floor henceforth; this will be the case if the rod does not bounce. Is it possible to place the rod in such a position that, if it is released at the instant when the train starts and allowed to move solely under the influence of gravity and the motion of the train, it will not fall to the floor during the entire journey from A to B?
The solution is elegant: introduce the assumption that the final angle of the rod depends continuously on the initial angle. The Intermediate-Value Theorem then shows that there is an initial position from which the rod does not hit the floor-in fact, one from which it ends up vertical or at any other specified angle.
Stewart writes in his preface that the viewpoint has changed over the years, so that while the argument just quoted is correct within the stated assumptions, these assumptions now seem less reasonable than they did back then. In his report on recent developments (p. 506), he maintains that the continuity assumption is "arguably not justified," referring an article by Tim Poston "Au Courant with differential equations" in Manifold (1976). (Poston's term is "wrong".)
But in order for paths to come arbitrarily near the floor without touching it and then swing back up, which Poston envisions, the acceleration of the train would have to be unbounded, which is not possible from a physical locomotive. The following proof that the final angle of the rod is a continuous function of the initial angle was contributed by my colleague Charles Radin.
We consider itineraries of the train x(t), t  [0,T], for which the horizontal acceleration is assumed to be bounded. Let V(t) be the downward component of the linear velocity of the end of the rod and H(t) the height of the rod from the floor, both at time t. First we prove:
For any given physical setup and itinerary x(t), there is an  > 0 such that, if H(t) < and V(t) is either zero or directed towards the floor, then H(t')  H(t) for t' < t < T [sic].
This follows almost immediately from noticing that when the rod is almost at the floor, the angular acceleration of the rod produced by the bounded horizontal accelerations of the train must be smaller in magnitude than the vertical acceleration caused by gravity; the net acceleration must then be directed toward the floor, and if the angular velocity is also directed toward the floor the conclusion follows.
To prove continuity of the function g that takes an initial angle of the rod to its final angle, we consider two cases: an initial angle  from which the rod (i) does not hit the floor, or (ii) does hit the floor, say at time 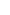 (and then stays on the floor).
Consider the modified motion that would be produced if the rod could rotate through a slot in the floor. This modified motion is correctly modeled by a standard differential equation that ensures that the modified angle of the rod at time t depends continuously on the initial angle.
Continuity for case (i) then follows because modified and unmodified motions are the same for initial angles sufficiently close to . For case (ii), we see by considering the modified motion that an initial angle very close to , either leads to hitting the floor very soon before 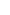, or else leads to a height very close to the floor (closer than the above ) at time 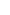, and heading toward the floor. In either case our analysis shows that the unmodified motion stays close to the floor for t > 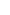, which means that we have continuity.
|
| « Last Edit: May 18th, 2007, 2:31pm by Eigenray » |
IP Logged |
|
|
|
towr
wu::riddles Moderator
Uberpuzzler
Some people are average, some are just mean.
Gender:
Posts: 13730
|
|
Re: Train Rod
« Reply #12 on: May 19th, 2007, 1:11pm » |
Quote Modify
|
Considering the train can stop in between, the rod would fall if at any stop it isn't perfectly upright. So stop, and after an appropriate wait, move a bit and stop again (with same acceleration). And after an other appropriately long wait the rod must be on the floor, because it will have been brought out of ballance while moving (and fell a bit, so it couldn't get back up during a stop with the some acceleration).
|
| « Last Edit: May 19th, 2007, 1:21pm by towr » |
IP Logged |
Wikipedia, Google, Mathworld, Integer sequence DB
|
|
|
Eigenray
wu::riddles Moderator
Uberpuzzler
Gender:
Posts: 1948
|
|
Re: Train Rod
« Reply #13 on: May 19th, 2007, 3:01pm » |
Quote Modify
|
on May 19th, 2007, 1:11pm, towr wrote:| Considering the train can stop in between, the rod would fall if at any stop it isn't perfectly upright. So stop, and after an appropriate wait, move a bit and stop again (with same acceleration). And after an other appropriately long wait the rod must be on the floor, because it will have been brought out of ballance while moving (and fell a bit, so it couldn't get back up during a stop with the some acceleration). |
|
But the time it takes the rod to fall is not bounded. That is, for any time T, there is an open interval of starting positions around vertical from which the rod will not hit the ground before T:
If the train is at rest, the offset from vertical satisfies something like '' = sin . Since sin < , it follows (t) < 0 cosh(t), so we need only take |0| <  /(2cosh T); then |(T)| < /2.
|
|
IP Logged |
|
|
|
SWF
Uberpuzzler
Posts: 879
|
|
Re: Train Rod
« Reply #14 on: May 19th, 2007, 7:38pm » |
Quote Modify
|
In the initial problem statement, claiming final position is a continuous function of initial postion is glossing over the crux of the problem. I don't think that is even right. Consider what happens if acceleration of the train, a(t), is sin(1/t) for t>0, and the rod starts in the neighborhood of the vertical position.
For a finite continuous a(t) with a finite number of local maxima and minima, I do think you can always make the rod be vertical at the end of the trip, and the differential equation is
d2w/dt2 + (3g/(2L))*cos(w) = (3/(2L))*a(t)*sin(w)
(t=time, L=length of rod, g=accel due to gravity, a(t)=acceleration of train vs. time, w=angle of rod)
To find the required starting point, try starting from the desired ending position and work backwards by cutting a(t) up into segments- each segment is either monotonic increasing or decreasing and has a local maximum or minimum at each end point. Call accelerations at end points of one of these steps a1 and a2, and angular positions at endpoints w1 and w2. Since we are working backwards, we know w2. Try to find a w1 with dw/dt=0 that ends up at w2 as acceleration goes from a1 to a2, and move on to the next step. If acceleration is increasing over one of these segments then w must be greater than arctan(g/a2) during the segment, and if acceleration is decreasing over a segment w<arctan(g/a2) throughout the segment.
|
|
IP Logged |
|
|
|
ThudnBlunder
wu::riddles Moderator
Uberpuzzler
The dewdrop slides into the shining Sea
Gender:
Posts: 4489
|
A nice exposition, Eigenray. I wasn't aware there had been further developments since Posten's original article in 1976.
on May 18th, 2007, 2:21pm, Eigenray wrote:
To elucidate, the graphs below show the histories of various initial positions. The two are identical except that in (b) the vertical lines represent the absorbing boundary conditions. The history shown as a bold line is OK in (a) as there are no boundary conditions. However, because in (b) it is tangent to the 180o boundary condition which represents the floor, it causes a discontinuity. Any initial position to the left of the bold line ends up on the floor at 0o and any initial position on or to the right of the bold line ends up at 180o. (According to Posten.)
|
| « Last Edit: May 20th, 2007, 12:32pm by ThudnBlunder » |
IP Logged |
THE MEEK SHALL INHERIT THE EARTH.....................................................................er, if that's all right with the rest of you.
|
|
|
Grimbal
wu::riddles Moderator
Uberpuzzler
Gender:
Posts: 7527
|
|
Re: Train Rod
« Reply #16 on: May 20th, 2007, 12:15pm » |
Quote Modify
|
It seems to me that you never need to pass the 0° or 180° limit.
The closer you get to 180°, the larger acceleration you need to possibly get the rod back to 90°. Assuming the acceleration is limited (or at least the integral of it over any time interval), whatever your situation, when you are close enough to 180°, you will fall to 180° before going back to 90°, same for 0°, so you will never need to venture below the horizontal to reach the target of 90° at the end station. It is not very formal, but you get the idea.
|
| « Last Edit: May 20th, 2007, 12:20pm by Grimbal » |
IP Logged |
|
|
|
SWF
Uberpuzzler
Posts: 879
|
|
Re: Train Rod
« Reply #17 on: May 20th, 2007, 6:41pm » |
Quote Modify
|
Those plots are incorrect. It is not possible for the curve come in tangent at 180 and turn around because the rod would be instantaneously stopped at 180 degrees with only a downward force compoent.
A more instructive plot might be to make the dark colored curve be arctan(g/a(t)) vs. time. Here, as in my previous post I mean arctan() to give an angle from 0 to 180, rather than the usual -90 to +90.
|
|
IP Logged |
|
|
|
rmsgrey
Uberpuzzler
Gender:
Posts: 2873
|
|
Re: Train Rod
« Reply #18 on: May 21st, 2007, 10:10am » |
Quote Modify
|
It may be helpful to think of the problem in terms of varying the direction (and intensity) of gravity rather than in terms of variable acceleration. Then again, it may not.
I can see two problems with the (a) graph shown:
1) As SWF pointed out, the curve tangent to 180 can only leave moving towards 270, and curves close enough to that will spend a very long time close to 180.
2) Rather than running roughly parallel, the curves should be converging on one point in 180-360 and diverging from its antipode in 0-180 at any given moment - with the possibility of convergent curves crossing - so at any given moment, there is a narrow fan of states that will diverge to cover 0-180 over a given time.
|
|
IP Logged |
|
|
|
SMQ
wu::riddles Moderator
Uberpuzzler
Gender:
Posts: 2084
|
|
Re: Train Rod
« Reply #19 on: May 21st, 2007, 1:23pm » |
Quote Modify
|
on May 21st, 2007, 10:10am, rmsgrey wrote:| Rather than running roughly parallel, the curves should be converging on one point in 180-360 and diverging from its antipode in 0-180 at any given moment |
|
Actually, because the natural tendency of the frictionless system is to either spin forever around the pivot (if there's enough kinetic energy), or oscillate about 270-degrees (if there's not), after just a little time you end up with paths crisscrossing chaotically in all directions.
I've been playing around in Excel and the only conclusion I've been able to draw definitively is that 1/1000 of a second isn't sufficient resolution for a numerical simulation to stabilize. 
--SMQ
|
|
IP Logged |
--SMQ
|
|
|
SWF
Uberpuzzler
Posts: 879
|
|
Re: Train Rod
« Reply #20 on: May 21st, 2007, 8:52pm » |
Quote Modify
|
True, about curves on a theta vs. time plot crossing. If two curves cross they have the same theta at that time, but d(theta)/dt could be different giving them a different past and future. For example, a rod starting at some angle can build more momentum that one starting at lower angle and overtake it at a later time.
Has anyone thought about the a(t)=sin(1/t) example given earlier?
|
|
IP Logged |
|
|
|
Barukh
Uberpuzzler
Gender:
Posts: 2276
|
|
Re: Train Rod
« Reply #21 on: May 22nd, 2007, 8:59am » |
Quote Modify
|
SWF's example describes a function that is not defined at t = 0. Moreover, it's derivative is not bounded.
Did I get it right that the discussion is about the conditions put on the acceleration function a(t) in order for question to be answered in affirmative?
|
|
IP Logged |
|
|
|
SMQ
wu::riddles Moderator
Uberpuzzler
Gender:
Posts: 2084
|
|
Re: Train Rod
« Reply #22 on: May 22nd, 2007, 10:49am » |
Quote Modify
|
on May 21st, 2007, 8:52pm, SWF wrote:| Has anyone thought about the a(t)=sin(1/t) example given earlier? |
|
Here are a couple illustrative plots of the region around 90-degrees (+/- ~20') for a rod of length 1.5m over the first ~4 seconds and a rod of length 1" over the first ~1/2 second, respectively, with a = sin(1/t) in gravities and seconds, using a discrete simulation with microsecond resolution. The colored lines start out ~10 arc seconds apart; the light gray lines are 0 and 180 degrees; the dark gray lines are relative "up" and "down" taking the acceleration into account.
 
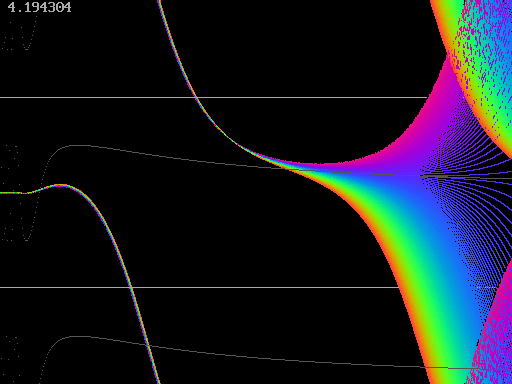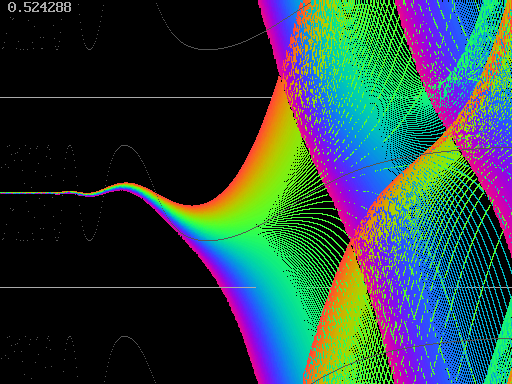
While the dispersion is certainly high, both plots would still appear to be continuous.
--SMQ
|
|
IP Logged |
--SMQ
|
|
|
SWF
Uberpuzzler
Posts: 879
|
|
Re: Train Rod
« Reply #23 on: May 23rd, 2007, 8:47pm » |
Quote Modify
|
on May 22nd, 2007, 8:59am, Barukh wrote:SWF's example describes a function that is not defined at t = 0. Moreover, it's derivative is not bounded.
Did I get it right that the discussion is about the conditions put on the acceleration function a(t) in order for question to be answered in affirmative?
|
|
The value of acceleration at t=0 should not matter as long as it is finite, but define it as zero there if you like. There is no requirement that deriviative of acceleration be bounded, and the motion depends on acceleration anyway, not its derivative. The point I am trying to make with sin(1/t) is that for the a(t) allowed by the problem statement, I don't think the final position is necessarily a continuous function of initial position (but I am not certain).
Those are nice plots, SMQ, but using finite sized time steps, you are skipping over the beginning of a(t) and will certainly get a continuous function that way. An interesting thing to plot would final position (say 1 second after start for the 1" long rod) vs. initial position and make several curves, each curve for a different size of time step. Stop when you hit 180 or 0 instead of plotting as if there are no barriers. If the curves are different, the time step is not small enough.
|
| « Last Edit: May 23rd, 2007, 8:49pm by SWF » |
IP Logged |
|
|
|
SMQ
wu::riddles Moderator
Uberpuzzler
Gender:
Posts: 2084
|
|
Re: Train Rod
« Reply #24 on: May 24th, 2007, 8:44am » |
Quote Modify
|
on May 23rd, 2007, 8:47pm, SWF wrote:| Those are nice plots, SMQ, but using finite sized time steps, you are skipping over the beginning of a(t) and will certainly get a continuous function that way. [...] If the curves are different, the time step is not small enough. |
|
Before posting them I did try the simulation at different time steps; an order of magnitude either way makes no visible difference.
I think that, near t = 0, the very short (approaching infinitesimal as t approaches 0) duration of the wildly varying acceleration limits its effect. That is, as the frequency of the acceleration increases it takes a smaller displacement (proportional to 1/f2) to achieve the same acceleration, so near t=0 the rod is being vibrated at very high frequency but very low amplitude -- which has a negligible effect on the dispersion of similar initial conditions.
It seems to me that any actual discontinuity would have to be the result of a(t) being undefined exactly at t = 0, since it's certainly continuous on (0, ].
--SMQ
|
|
IP Logged |
--SMQ
|
|
|
|
RIDDLES SITE
WRITE MATH!
Home 
Help 
Search 
Members 
Login 
Register
Reply
Notify of replies
Send Topic
Print