 Author
Author |
Topic: An Astute Sequence Puzzle (Read 531 times) |
|
K Sengupta
Senior Riddler
   

Gender: 
Posts: 371
|
 |
An Astute Sequence Puzzle
« on: Feb 16th, 2007, 12:05am » |
 Quote Quote  Modify Modify
|
Consider the sequence {a]n; n>0} defined by a0 = 0 and :
an+1 = [(an+ n)1/3 ]3 for n>=0; [x] is the greatest integer less than or equal to x.
(A) Determine an explicit formula representing an as a function of n only.
(B) Determine all n such that an = n
|
|
 IP Logged IP Logged |
|
|
|
Eigenray
wu::riddles Moderator
Uberpuzzler
    

Gender: 
Posts: 1948
|
 |
Re: An Astute Sequence Puzzle
« Reply #2 on: Feb 24th, 2007, 8:32pm » |
 Quote Quote  Modify Modify
|
To finish this off:
Say an = k3. Then an+1 = k3 as well, unless
(k3+n)1/3 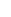 k+1. k+1.
i.e., n 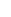 f(k) = (k+1)3 - k3. So if n<f(k), we have an+1 = k3 = an, and once n=f(k), we have an+1 = (k+1)3. f(k) = (k+1)3 - k3. So if n<f(k), we have an+1 = k3 = an, and once n=f(k), we have an+1 = (k+1)3.
It follows that for k>0, if f(k-1) < n  f(k), then an = k3. f(k), then an = k3.
So in order to have n = an = k3 >0, we need f(k-1) < k3  f(k). But k3 f(k). But k3  (k+1)3 - k3 when 2k3 (k+1)3 - k3 when 2k3  (k+1)3, or 1+1/k (k+1)3, or 1+1/k 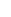 21/3 ~ 1.26, which requires k 21/3 ~ 1.26, which requires k  3. Checking these, we find only n = 0, 8, and 27 work. 3. Checking these, we find only n = 0, 8, and 27 work.
|
|
 IP Logged IP Logged |
|
|
|
|
 WRITE MATH!
WRITE MATH!
 Home
Home  Help
Help  Search
Search  Members
Members  Login
Login  Register
Register WRITE MATH!
WRITE MATH!
 Home
Home  Help
Help  Search
Search  Members
Members  Login
Login  Register
Register