Author |
Topic: square to pentomino (Read 880 times) |
|
JocK
Uberpuzzler
Gender: 
Posts: 877
|
 |
square to pentomino
« on: Feb 5th, 2005, 2:39pm » |
 Quote  Modify
|
Pentominos are the connected planar rectangular shapes that originate when arranging five squares of equal size such that each square has at least one side coincident with another.
Can you cut a square into pieces such that the pieces can be arranged* into any pentomino (of the same area as the square)? What is the minimum number of pieces you need?
* translations, rotations and flipping of the pieces are all allowed
|
| « Last Edit: Feb 6th, 2005, 2:04am by JocK » |
 IP Logged |
solving abstract problems is like sex: it may occasionally have some practical use, but that is not why we do it.
xy - y = x5 - y4 - y3 = 20; x>0, y>0.
|
|
|
rmsgrey
Uberpuzzler
    
Gender:
Posts: 2873
|
|
Re: square to pentomino
« Reply #1 on: Feb 5th, 2005, 6:20pm » |
Quote Modify
|
on Feb 5th, 2005, 2:39pm, JocK wrote:| Pentominos are the rectangular shapes that originate when arranging five squares of equal size such that each square has at least one side coincident with another. |
|
There's only one rectangular pentomino...
Without the rectangular requirement, your definition includes all the shapes you can make by randomly placing a domino and a tromino in the plane,as well as various non-planar shapes.
An alternate way of defining them is: "the shapes you can make by picking 5 orthogonally connected squares on a chessboard"
|
|
IP Logged |
|
|
|
SWF
Uberpuzzler
Posts: 879
|
|
Re: square to pentomino
« Reply #2 on: Feb 5th, 2005, 7:17pm » |
Quote Modify
|
The pentomino in the shape of a "+" cannot be made from a domino and a triomino.
One easy way, but perhaps not with the minimum piece count: Cut out two square pieces and a domino. The remainder easily be can be cut into pieces that form a rectangle. The rectangle is pretty simple to cut so it can form a square. The 3 squares and a domino can make all the required shapes..
|
|
IP Logged |
|
|
|
rmsgrey
Uberpuzzler
Gender:
Posts: 2873
|
|
Re: square to pentomino
« Reply #3 on: Feb 5th, 2005, 8:04pm » |
Quote Modify
|
on Feb 5th, 2005, 7:17pm, SWF wrote:| The pentomino in the shape of a "+" cannot be made from a domino and a triomino. |
|
on Feb 5th, 2005, 6:20pm, rmsgrey wrote:
Without the rectangular requirement, your definition includes all the shapes you can make by randomly placing a domino and a tromino in the plane, as well as various non-planar shapes. |
|
So how many planar shapes which are not pentominoes fit JocK's definition but cannot be made up of a domino and a tromino?
|
|
IP Logged |
|
|
|
JocK
Uberpuzzler
Gender:
Posts: 877
|
|
Re: square to pentomino
« Reply #4 on: Feb 6th, 2005, 1:53am » |
Quote Modify
|
on Feb 5th, 2005, 6:20pm, rmsgrey wrote:
There's only one rectangular pentomino...
Without the rectangular requirement, your definition includes all the shapes you can make by randomly placing a domino and a tromino in the plane,as well as various non-planar shapes. |
|
OK, OK, ... 
Will update the 'definition'...
on Feb 5th, 2005, 6:20pm, rmsgrey wrote:
An alternate way of defining them is: "the shapes you can make by picking 5 orthogonally connected squares on a chessboard" |
|
Are you sure that if I use this definition here I will not be asked the question "Define chessboard" ... ?
|
| « Last Edit: Feb 6th, 2005, 1:57am by JocK » |
IP Logged |
solving abstract problems is like sex: it may occasionally have some practical use, but that is not why we do it.
xy - y = x5 - y4 - y3 = 20; x>0, y>0.
|
|
|
Grimbal
wu::riddles Moderator
Uberpuzzler
Gender:
Posts: 7527
|
|
Re: square to pentomino
« Reply #5 on: Feb 7th, 2005, 2:38am » |
Quote Modify
|
Whatever the definition, here is a list of all possible pentominoes.
http://www.theory.csc.uvic.ca/~cos/inf/misc/PentInfo.html
It is already an interesting question how to cut a square in a minimum number of pieces to make each of the pentominoes individually. But here, it seems we have to come up with a single set that will recombine into any one of the pentominoes.
I have a solution in 5 pieces. ::
#####################
#__####____________##
#______####_______#_#
#__________####__#__#
#______________##___#
###____________#____#
#__####_______#_____#
#__#___####__#______#
#_#________####_____#
##_____________####_#
#####################
::
|
| « Last Edit: Feb 7th, 2005, 2:39am by Grimbal » |
IP Logged |
|
|
|
JocK
Uberpuzzler
Gender:
Posts: 877
|
|
Re: square to pentomino
« Reply #6 on: Feb 7th, 2005, 3:24pm » |
Quote Modify
|
If this works it beats my own solution.... 
But... are you sure you can create all 12 pentominos from these five pieces? How would you rearrange the five pieces into a 5x1 bar?
|
|
IP Logged |
solving abstract problems is like sex: it may occasionally have some practical use, but that is not why we do it.
xy - y = x5 - y4 - y3 = 20; x>0, y>0.
|
|
|
Grimbal
wu::riddles Moderator
Uberpuzzler
Gender:
Posts: 7527
|
|
Re: square to pentomino
« Reply #7 on: Feb 8th, 2005, 1:07am » |
Quote Modify
|
Oops....
Don't you want me to do the W instead? 
Actually the + would also be a problem.
|
|
IP Logged |
|
|
|
SWF
Uberpuzzler
Posts: 879
|
Excuse me, rmsgrey, I thought you were suggesting a clue to the solution rather than pointing out an error in the phrasing of the question.
The following uses 7 pieces (pieces of the same color pair up to form either a domino or a square, 3 squares and a domino can make any pentomino):
|
|
IP Logged |
|
|
|
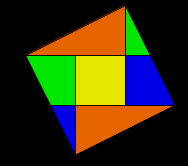
JocK
Uberpuzzler
Gender:
Posts: 877
|
|
Re: square to pentomino
« Reply #9 on: Feb 9th, 2005, 3:03pm » |
Quote Modify
|
Yes, cutting two of Grimbal's pieces does the job. As long as Barukh doesn't post a solution with fewer pieces this is the optimum. 
Well done SWF.
|
|
IP Logged |
solving abstract problems is like sex: it may occasionally have some practical use, but that is not why we do it.
xy - y = x5 - y4 - y3 = 20; x>0, y>0.
|
|
|
|
RIDDLES SITE
WRITE MATH!
Home 
Help 
Search 
Members 
Login 
Register
Reply
Notify of replies
Send Topic
Print