Author |
Topic: Two envelopes problem - a variation (Read 11083 times) |
|
Altamira_64
Junior Member
Posts: 116
|
 |
Two envelopes problem - a variation
« on: Jun 17th, 2012, 5:42am » |
 Quote  Modify
|
This is a variation to the known problem, with both parties knowing their amount and also the possible amounts being within predefined boundaries:
Your grandpa gives one envelope with a checque to you and your sister Christine and tells you that the amount can be anything from $5 to $160 and that one checque has double the amount of the other. You take a secret look to your own checque inside the envelope and see that it's $10. Your sister does the same. Grandpa tells you that if you want, you have the option to swap your envelopes, provided you both agree.
Would you go for swapping or not? Take into consideration the following 2 clarifications:
It is equally likely for each checque to have half or double the amount of the other.
Your decision to swap or keep your checque must be secretely told to your grandpa, without the other party knowing each other's decision.
|
|
 IP Logged |
|
|
|
towr
wu::riddles Moderator
Uberpuzzler
Some people are average, some are just mean.
Gender: 
Posts: 13730
|
|
Re: Two envelopes problem - a variation
« Reply #1 on: Jun 17th, 2012, 6:45am » |
Quote Modify
|
If it's 5 try to swap, if it's 160 don't.
Since the person with 160 isn't going to swap (no where to go but down), the person with 80 would only swap with the person with 40. So the person with 80 shouldn't swap either.
For similar reasons, in general: the person with 2x would only swap with the person with x; so never swap unless you have the lowest amount. In which case, don't bother.
|
| « Last Edit: Jun 17th, 2012, 6:46am by towr » |
IP Logged |
Wikipedia, Google, Mathworld, Integer sequence DB
|
|
|
Altamira_64
Junior Member
Posts: 116
|
|
Re: Two envelopes problem - a variation
« Reply #2 on: Jun 17th, 2012, 11:55am » |
Quote Modify
|
"the person with 80 would only swap with the person with 40. So the person with 80 shouldn't swap either"
Yes but how does the person with 80 know that the other person has 40 and not 160? How do we deduce that he would not go for swapping?
Keep in mind that each person tells his decision (to swap or keep) secretely, so the other person's intention is not known yet.
|
|
IP Logged |
|
|
|
towr
wu::riddles Moderator
Uberpuzzler
Some people are average, some are just mean.
Gender:
Posts: 13730
|
|
Re: Two envelopes problem - a variation
« Reply #3 on: Jun 17th, 2012, 1:08pm » |
Quote Modify
|
She doesn't know that the other person doesn't have 160, but she knows the person with 160 would not swap, because for him the only way is down. The only person that might swap would have 40, so she'd lose by swapping with him. The puzzle stipulates both have to agree to swap.
If the other would only ever agree to swap when he has a lower amount, then you shouldn't swap.
So either she has 80 and the other has 160, she swaps, he doesn't, she keeps 80.
Or the other person has 40, she swaps, he swaps, she get's 40 instead of 80, i.e. a net loss of 40.
And the reasoning runs all the way down from the top, so if there's an upper limit there is never a reason to swap, because the person with double the amount as you wouldn't swap.
Which is why the original "two envelope problem" were there isn't an upper limit "works".
|
| « Last Edit: Jun 17th, 2012, 1:12pm by towr » |
IP Logged |
Wikipedia, Google, Mathworld, Integer sequence DB
|
|
|
Altamira_64
Junior Member
Posts: 116
|
|
Re: Two envelopes problem - a variation
« Reply #4 on: Jun 17th, 2012, 3:07pm » |
Quote Modify
|
Yes, but note the last assumption:
"Your decision to swap or keep your checque must be secretely told to your grandpa, without the other party knowing each other's decision".
Yes, they must both agree, but each of them tells grandpa whether he/she will swap or keep, without knowing what the other has decided.
|
|
IP Logged |
|
|
|
towr
wu::riddles Moderator
Uberpuzzler
Some people are average, some are just mean.
Gender:
Posts: 13730
|
|
Re: Two envelopes problem - a variation
« Reply #5 on: Jun 17th, 2012, 10:19pm » |
Quote Modify
|
That doesn't matter. If you have 160, then I know without you telling me that in that case you wouldn't swap. Not if you are in any way rational. If you have the highest amount you can get, then you wouldn't swap. If you have 160, you won't want to swap, because the only way is down. If you have 80, then the only way is down, because if the other had 160 she wouldn't want to swap, so therefor you shouldn't swap. If you have 40, you won't want to swap because if the other person had 80 he wouldn't want to swap, so you can never gain anything, only lose. If you have 20, you won't want to swap, because the person with 40 wouldn't.
Why would anyone swap if they only stand to lose? Is my opponent an idiot? Because then I'd swap as long as I don't have 160. But if she's in any way rational and capable of reasoning, then I wouldn't swap, because there is nothing to win and only anything to lose (except when I already have the lowest amount, but then she wouldn't swap anyway).
|
| « Last Edit: Jun 17th, 2012, 10:20pm by towr » |
IP Logged |
Wikipedia, Google, Mathworld, Integer sequence DB
|
|
|
Altamira_64
Junior Member
Posts: 116
|
|
Re: Two envelopes problem - a variation
« Reply #6 on: Jun 18th, 2012, 3:39am » |
Quote Modify
|
on Jun 17th, 2012, 10:19pm, towr wrote:| If you have 80, then the only way is down, because if the other had 160 she wouldn't want to swap, so therefor you shouldn't swap. |
|
OK: If you have 80, you don't know what the other has. Maybe he has 160 but you don't know it by the time you (secretly) tell grandpa that you want to swap. You must first tell grandpa your decision and afterwards grandpa will tell you what your sister has decided. Your sister also (secretely) tells grandpa if she wants to swap or not, without knowing your decision!
|
|
IP Logged |
|
|
|
towr
wu::riddles Moderator
Uberpuzzler
Some people are average, some are just mean.
Gender:
Posts: 13730
|
|
Re: Two envelopes problem - a variation
« Reply #7 on: Jun 18th, 2012, 8:47am » |
Quote Modify
|
I can't for the live of me fathom why you don't understand this.
If I have 80, there are two options:
Either I have 80 and the other person has 160, or I have 80 and the other person has 40. Correct or not?
If the other person has 160, then she has the highest amount she can get, and therefor she know that I have 80, so she will not want to share. Correct or not?
If the other person has 40, quite frankly, I'm not very interested if she will want to share or not, because I have nothing to gain and much to lose. Correct or not?
So either I want to share but she won't let me, or she might want to share and I shouldn't let her because I can only lose. In neither case should we both agree to share if we're both rational people with even a very limited capacity to think about what other people think.
If you still don't believe it, I would very much like to play this game with you a few hundred games, cause I can always use the extra money.
Or you could run a simulation.
|
| « Last Edit: Jun 18th, 2012, 8:50am by towr » |
IP Logged |
Wikipedia, Google, Mathworld, Integer sequence DB
|
|
|
Grimbal
wu::riddles Moderator
Uberpuzzler
Gender:
Posts: 7527
|
|
Re: Two envelopes problem - a variation
« Reply #8 on: Jun 18th, 2012, 12:29pm » |
Quote Modify
|
I do agree with towr.
To elaborate on towr's answer:
If towr plays the game, he will propose a swap only when he finds an amount below $10. He will keep his envelope otherwise.
This way he is certain he will never loose money in a swap. If you play against him, you are certain never to win anything when proposing to swap. So you might as well never offer to swap.
|
|
IP Logged |
|
|
|
Altamira_64
Junior Member
Posts: 116
|
|
Re: Two envelopes problem - a variation
« Reply #9 on: Jan 20th, 2013, 4:04am » |
Quote Modify
|
Here is another variation:
Two players, A and B, are going to play a game. A perfect logician explains the terms:
I have several envelopes containing different amounts of money. I will randomly pick one of them, see the amount that it contains and will give it closed to player A. Then I toss a coin and if I get tails, I will get an empty envelope and put half the amount of player's A envelope, while if I get heads, will put double. I will then give this envelope (closed) to player B.
Then I will invite each of you privately and ask you to decide whether you will swap envelopes or not. If you both agree in swapping, you will do so, otherwise you will keep your initial envelopes.
A and B agree with the procedure and then A asks B to reveal his amount, so that they get an idea on what to propose to the logician. They both see that B has $100. Right afterwards, each of them must meet the logician to announce their decision. Which decision ensures the biggest expected gain for players A and B separately? Explain your answer.
|
|
IP Logged |
|
|
|
towr
wu::riddles Moderator
Uberpuzzler
Some people are average, some are just mean.
Gender:
Posts: 13730
|
|
Re: Two envelopes problem - a variation
« Reply #10 on: Jan 20th, 2013, 8:23am » |
Quote Modify
|
A should want to switch and B shouldn't.
For any amount X that A gets there's 50-50 chance B gets either 2X or 1/2X, so on average A would gain from switching and by symmetry (as you'd expect) B would lose.
|
|
IP Logged |
Wikipedia, Google, Mathworld, Integer sequence DB
|
|
|
Altamira_64
Junior Member
Posts: 116
|
|
Re: Two envelopes problem - a variation
« Reply #11 on: Jan 24th, 2013, 10:56am » |
Quote Modify
|
So, how does B know that he will lose by swapping?
He knows that A has either $50 or $200, so by swapping he has more to gain than to lose.
|
|
IP Logged |
|
|
|
towr
wu::riddles Moderator
Uberpuzzler
Some people are average, some are just mean.
Gender:
Posts: 13730
|
|
Re: Two envelopes problem - a variation
« Reply #12 on: Jan 24th, 2013, 10:08pm » |
Quote Modify
|
on Jan 24th, 2013, 10:56am, Altamira_64 wrote:| He knows that A has either $50 or $200, so by swapping he has more to gain than to lose. |
|
You don't know that, because it depends on the a priori probability of A getting a $50 or $200 bill. If there aren't any $200 bills on the table to start with, then B hasn't even a chance to gain.
Since you don't know the prior distribution any inference based on the observation that B has $100 is meaningless.
|
|
IP Logged |
Wikipedia, Google, Mathworld, Integer sequence DB
|
|
|
roady
Newbie
Gender:
Posts: 2
|
|
Re: Two envelopes problem - a variation
« Reply #13 on: Feb 26th, 2013, 12:52pm » |
Quote Modify
|
The thing is that even though you know that A can have 1/2x or 2x given that B has X. the probability of it being either of those possibilities is NOT 50/50.
(So even though the chances of B given A are 50/50, the chances of A given B don't need to be 50/50 per sé)
In fact the observation that B has x adds no useful information for influencing A his decision.
|
|
IP Logged |
|
|
|
antkor
Newbie
Posts: 30
|
|
Re: Two envelopes problem - a variation
« Reply #14 on: Dec 16th, 2013, 4:46pm » |
Quote Modify
|
on Jan 20th, 2013, 8:23am, towr wrote:A should want to switch and B shouldn't.
For any amount X that A gets there's 50-50 chance B gets either 2X or 1/2X, so on average A would gain from switching and by symmetry (as you'd expect) B would lose. |
|
Could you analyze that a bit more? Perhaps giving some mathematic proof?
|
|
IP Logged |
|
|
|
towr
wu::riddles Moderator
Uberpuzzler
Some people are average, some are just mean.
Gender:
Posts: 13730
|
|
Re: Two envelopes problem - a variation
« Reply #15 on: Dec 16th, 2013, 10:26pm » |
Quote Modify
|
on Dec 16th, 2013, 4:46pm, antkor wrote:| Could you analyze that a bit more? Perhaps giving some mathematic proof? |
|
?
EEnvB= 0.5*EnvA*2 + 0.5*EnvA/2 = 1.25 EnvA
EnvA < EEnvB, therefore A should want to switch and B shouldn't.
|
|
IP Logged |
Wikipedia, Google, Mathworld, Integer sequence DB
|
|
|
EdwardSmith
Junior Member
Posts: 61
|
|
Re: Two envelopes problem - a variation
« Reply #16 on: Jul 17th, 2014, 10:13am » |
Quote Modify
|
Am I wrong again or is everybody else wrong.
No calculations are needed.
"You take a secret look to your own checque inside the envelope and see that it's $10."
You are told what your amount is.
This means you have a chance of gaining $10 or losing $5.
The only other bit of information you can take into account is that is
"the amount can be anything from $5 to $160"
therefore your sisters cheque has more chance of being a higher amount.
Take the swap.
|
|
IP Logged |
Schottland Pension
|
|
|
towr
wu::riddles Moderator
Uberpuzzler
Some people are average, some are just mean.
Gender:
Posts: 13730
|
Have you read the red-eyed monks puzzle? Because this is like that one. The problem is you have to consider a lot of possible-but-not-actual situations.
1) If you have $10, then you know that your sister has either $5 or $20
2) * If your sister has $5 dollar she knows you have $10 dollar, and will want to swap. And if you knew she had $5 dollar you would not want to swap.
3) * If your sister has $20, then she'll know that either you have $10 or $40
4) * * If you have $10 (which is actually the case, but your sister doesn't know this), then we're actually back at step 1, so I needn't have included it here again.
5) * * If you have $40, then you know that your sister either has $20 (goto step 3) or $80
6) * * * If she has $80, then she knows you have either $40 (goto step 5) or $160
7) * * * * If you have $160, then you don't want to swap, because there's no higher amount, though she'd be glad to swap.
* * * revisiting step 6: since you wouldn't swap if you had $160, she won't gain anything by proposing a swap and just set herself up to lose should you have $40. So she won't swap.
* * revisiting step 5: since she won't swap if she has $80, your just setting yourself up to lose if you propose to swap when you have $40, so you shouldn't.
* revisiting step 3: your sister isn't a sucker, by now she'll have realized you wouldn't swap if you had $40, because she wouldn't at $80, because you wouldn't at $160. So she won't swap when she has $20.
revisiting step 1: your sister will only propose a swap if she has $5, cause that's the only case where she has nothing to lose and everything to gain. So in conclusion you shouldn't swap.
Now, the tricky bit for most people is realizing that the you's and she's from step 2 onward are hypothetical you's and she's, because obviously if you have $10 you know 5,6,7 are not the case. However, to the hypothetical sister in step 3) the you in step 5) is a hypothetical possibility, even though it isn't to you in step 1). You have to work through the whole graph of hypotheticals to get at the correct answer.
Or just consider that you should never swap anything with someone that values them the same as you. (Because in that case you can't both be better off.)
As we say in the Netherlands "Van ruilen komt huilen" (swapping leads to tears).
There's also a graphical solution, maybe I'll add that tomorrow.
[edit]
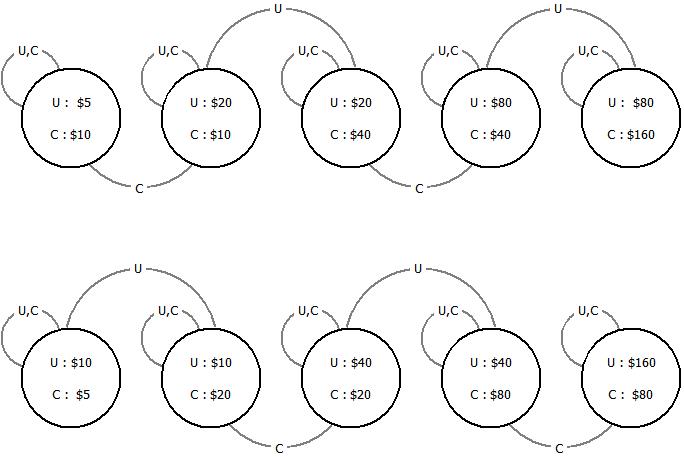
I've attached a model of problem below, covering all possible states you (U) and your sister (C) could find yourself in, and how those states relate to each other.
The connecting lines denote which state you'd think are possible state you might be in given the one your actually in. So for example if you're in state U:$10,C:$5, then (because you don't know what your sister has) it's possible you're either in U:$10,C:$5 or U:$10,C:$20.
Now it's just a matter of filling in for each state how you and she should decide given the knowledge in that state. And the states to start with is where someone has full knowledge (i.e. no doubt about which state they are in).
These are states U:$10,C:$5, U:$5,C:$10, U:$80,C:$160, U:$160,C:$80; the person with $160 will know they don't want to swap, and the person with $5 does want to swap.
So now state U:$160,C:$80 becomes U:$160[won't swap],C:$80, and U:$80,C:$160 becomes U:$80,C:$160[won't swap]
A person with $80 now has extra knowledge to consider. You might be in U:$80,C:$160[won't swap] or U:$80,C:$40, and regardless of whether your sister in the later state might want to swap or not, it's not a good proposition for you, because you know she wouldn't swap if she had $160. So states U:$80,C:$160[won't swap] and U:$80,C:$40 should be updated to U:$80[won't swap],C:$160[won't swap] and U:$80[won't swap],C:$40
And so on.
With the end result that only a person with $5 should propose swapping.
[/edit]
[e2]fixed error pointed out by rloginunix below.[/e2]
|
| « Last Edit: Jul 19th, 2014, 1:03pm by towr » |
IP Logged |
Wikipedia, Google, Mathworld, Integer sequence DB
|
|
|
rloginunix
Uberpuzzler
Posts: 1029
|
|
Re: Two envelopes problem - a variation
« Reply #18 on: Jul 19th, 2014, 12:56pm » |
Quote Modify
|
(Tiny typo in [edit] section, 3-rd paragraph beginning with "So now state": instead of "and U:$160,C:$80 becomes" you meant "and U:$80,C:$160 becomes")
I think towr should feel absolutely safe swapping the moderator and the explainer of things roles,  .
|
|
IP Logged |
|
|
|
towr
wu::riddles Moderator
Uberpuzzler
Some people are average, some are just mean.
Gender:
Posts: 13730
|
|
Re: Two envelopes problem - a variation
« Reply #19 on: Jul 19th, 2014, 1:42pm » |
Quote Modify
|
Thanks, both for the correction and the compliment.
Yet another way to explain it:
You can modify the problem so that you're the second youngest of 6 siblings, and grandpa's lined you all up from youngest to oldest and gives you $5,$10,$20,$40,$80,$160 respectively. Like before each sibling then gets to choose whether they're willing to swap (with an adjacent sibling but without the choice which). Also like before, the choices are kept secret from any other sibling and only shared with grandpa. And finally, when all choices are made, grandpa will randomly pick* two adjacent siblings that are willing to swap, and those two will swap their money.
And my claim is that this is actually equivalent to the original problem but without hypothetical states to consider.
*) the random pick here is solely because you can't swap your amount to both sides should your adjacent siblings by some fit of lunacy both be willing to.
|
| « Last Edit: Jul 19th, 2014, 1:48pm by towr » |
IP Logged |
Wikipedia, Google, Mathworld, Integer sequence DB
|
|
|
dudiobugtron
Uberpuzzler
Posts: 735
|
|
Re: Two envelopes problem - a variation
« Reply #20 on: Jul 20th, 2014, 5:24pm » |
Quote Modify
|
Imagine a slight alteration to the puzzle as follows:
Each of you writes on a piece of paper, either 'swap' or 'don't swap', and gives this to your grandfather in secret. The Grandfather then pulls one of these pieces of paper from his hat at random, to decide whether you will swap or not.
What should you write on your piece of paper?
|
|
IP Logged |
|
|
|
gregpap
Newbie
Posts: 4
|
|
Re: Two envelopes problem - a variation
« Reply #21 on: Aug 21st, 2014, 4:30am » |
Quote Modify
|
on Dec 16th, 2013, 10:26pm, towr wrote:
?
EEnvB= 0.5*EnvA*2 + 0.5*EnvA/2 = 1.25 EnvA
EnvA < EEnvB, therefore A should want to switch and B shouldn't. |
|
This is the solution, as the expected return is higher that 1, you should always change, unless you have the $160 check, where the expected return is negative already....
I don't believe this riddle should be in the hard category as it's very easy...cheers
|
|
IP Logged |
|
|
|
rmsgrey
Uberpuzzler
    
Gender:
Posts: 2873
|
|
Re: Two envelopes problem - a variation
« Reply #22 on: Aug 22nd, 2014, 7:07am » |
Quote Modify
|
on Aug 21st, 2014, 4:30am, gregpap wrote:
This is the solution, as the expected return is higher that 1, you should always change, unless you have the $160 check, where the expected return is negative already....
I don't believe this riddle should be in the hard category as it's very easy...cheers |
|
But if I'm playing against that strategy, then my expected value for switching the $80 check is negative - I either lose $40 or gain nothing because you refuse to switch with me. so if I know my opponent is using your "solution", I can make a profit by always ending up with the $80 when it's $40 and $80 in the envelopes (for any other pair, either we always switch and I win half; lose half, or we never switch, and again, I win half; lose half)
|
|
IP Logged |
|
|
|
Grimbal
wu::riddles Moderator
Uberpuzzler
Gender:
Posts: 7527
|
|
Re: Two envelopes problem - a variation
« Reply #23 on: Aug 22nd, 2014, 9:52am » |
Quote Modify
|
Actually the condition "the amount can be anything from $5 to $160" comes from the initial question, while towr's answer was to the alternate problem in reply #9. It is not clear whether the limit applies to the second problem.
Rmsgrey's answer refers to the original problem where you end up swapping only with a value <10.
Towr's answers to the alternate problem where A is selected randomly and B calculated from A. In that case, A should always switch and B never.
|
| « Last Edit: Aug 22nd, 2014, 9:53am by Grimbal » |
IP Logged |
|
|
|
rmsgrey
Uberpuzzler
Gender:
Posts: 2873
|
|
Re: Two envelopes problem - a variation
« Reply #24 on: Aug 23rd, 2014, 8:38am » |
Quote Modify
|
on Jul 20th, 2014, 5:24pm, dudiobugtron wrote:Imagine a slight alteration to the puzzle as follows:
Each of you writes on a piece of paper, either 'swap' or 'don't swap', and gives this to your grandfather in secret. The Grandfather then pulls one of these pieces of paper from his hat at random, to decide whether you will swap or not.
What should you write on your piece of paper? |
|
Assuming that all 5 possible pairs of envelopes are equally likely, and that you're equally likely to get either of a pair, you should write "swap" unless you get the $160 envelope.
The key difference here is that the actual outcome only depends on what you write or on what your sister writes, not on what both of you write - what you write only counts if your paper gets picked, in which case your sister's choice is irrelevant, so there's no need to take account of what your sister knows.
|
|
IP Logged |
|
|
|
|
RIDDLES SITE
WRITE MATH!
Home 
Help 
Search 
Members 
Login 
Register
Reply
Notify of replies
Send Topic
Print