Author |
Topic: Construct Medians (Read 8508 times) |
|
ThudnBlunder
wu::riddles Moderator
Uberpuzzler
The dewdrop slides into the shining Sea
Gender: 
Posts: 4489
|
 |
Construct Medians
« on: Sep 11th, 2003, 10:27am » |
 Quote  Modify
|
Construct the medians of a triangle with a straightedge alone, given that the vertices, taken pairwise, are foci to three given ellipses.
Hint: Ah, giving up already?? Sorry, I don't know the answer myself. 
|
| « Last Edit: Dec 12th, 2003, 3:16am by ThudnBlunder » |
 IP Logged |
THE MEEK SHALL INHERIT THE EARTH.....................................................................er, if that's all right with the rest of you.
|
|
|
Lightboxes
Full Member
Gender:
Posts: 203
|
|
Re: Construct Medians
« Reply #1 on: Oct 30th, 2003, 6:07pm » |
Quote Modify
|
I would like to take a stab at the puzzle...
but I'm not sure what some of it means?
Quote:given that the vertices, taken pairwise,
are foci to three given ellipses.
|
|
And when it says...
Quote:| Construct the medians of a triangle with a straightedge alone |
|
It is like finding the center of a line using a compass? You know, what students do in highschool.
|
|
IP Logged |
A job is not worth doing unless it's worth doing well.
|
|
|
Icarus
wu::riddles Moderator
Uberpuzzler
Boldly going where even angels fear to tread.
Gender:
Posts: 4863
|
|
Re: Construct Medians
« Reply #2 on: Oct 30th, 2003, 7:07pm » |
Quote Modify
|
One way to look at it is this: you are given a diagram consisting of 3 points (the vertices of the triangle), and three ellipses - each of which has as foci 2 of the points. The ellipses are not necessarily of the same eccentricity (it would be easier if they were).
You have no compass, only a straightedge. Using it you must find the midpoints of the triangle's 3 sides.
|
|
IP Logged |
"Pi goes on and on and on ...
And e is just as cursed.
I wonder: Which is larger
When their digits are reversed? " - Anonymous
|
|
|
Lightboxes
Full Member
Gender:
Posts: 203
|
Here is a template to play with if anyone wants to.
|
|
IP Logged |
A job is not worth doing unless it's worth doing well.
|
|
|
Lightboxes
Full Member
Gender:
Posts: 203
|
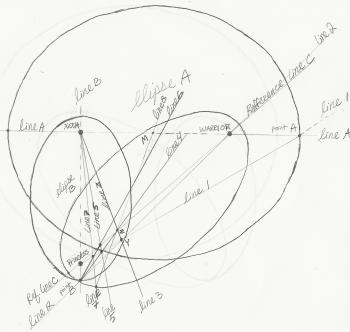
I used some dental floss and thumb tacks along with a carboard as a way to hold the tacks in place. Then I drew random elipses with random points as foci. Then I said to myself, "self, what lines can I draw, that have not been drawn yet, yet will help me find the median." So I decided that points A, B, and C were going to be helpful because those points are available (with certainty) in any triangle with three elipses (point C was not drawn because of its irrelevence in this particular drawing).
Then I drew lines that will intersect in any triangle given three elipses. Lines 1 and 2, I believe, will give that intersection of Y. Then using Reference line C (Line A, Line B, and Reference line C represent the triangle sides of triangle Xena Warrior Princess)
I came up with this picture by first drawing lines 1 and 2. Then lines 3 to 8 in order to get the median. Draw line one and 2 for an intersection. Then draw line 3 from Y to Xena, creating point Z. Then draw line 4 from B to the newly made intersection. Draw line 5 from the newly made intersection to Xena. Then line 6 from B to the newly made intersection. Then line 7 from the newly made intersection to Xena. Then line 8 from the edge of the elipse,intersecting line 7 to point Z, leading to the Median. (To summarize, these lines 3-7 are alternating from B to Xena). This also seemed to work with the other median of the same drawing. The other median was Xena Princess. This drawing is Median Xena Warrior.
NOTE: I'm still working on a proof. But I defintely wanted to post this because I know that other members of this forum are better at proofs than me.
|
|
IP Logged |
A job is not worth doing unless it's worth doing well.
|
|
|
Barukh
Uberpuzzler
Gender:
Posts: 2276
|
|
Re: Construct Medians
« Reply #5 on: Dec 16th, 2003, 11:32pm » |
Quote Modify
|
Lightboxes, your construction is very intriguing… Probably the most straightforward way to verify it would be to introduce the Cartesian coordinates and perform all these ugly computations. But before, I would like to ensure I read your drawing correct. Here’s how I got it (points 5-8 are not shown in your figure):
Line A: Points Xena & Warrior
Line B: Points Xena & Princess
Line C: Points Warrior & Princes
Point A: Intersection lineA & Ellipse A
Point B: Intersection line B & Ellipse B
Line 1: Points A and Princess
Line 2: Points B and Warrior
Point Y: Intersection lines 1 & 2
Line 3: Points Y & Xena
Point Z: Intersection lines 3 & C
Line 4: Points Z & B
Point 5: Intersection lines 4 & C
Line 5: Point 5 & Xena
Point 6: Intersection lines 5 & C
Line 6: Points 6 & B
Point 7: Intersection lines 6 & C
Line 7: Points 7 & Xena
Point 8: Intersection line 7 & Ellipse C
Line 8: Points 8 & Z
Point M: Intersection lines 8 & A
|
|
IP Logged |
|
|
|
Lightboxes
Full Member
Gender:
Posts: 203
|
|
Re: Construct Medians
« Reply #6 on: Dec 20th, 2003, 8:37pm » |
Quote Modify
|
I'm glad you have not done any work yet. I tried to get the median of the "hypothenus" but it didn't work. I think I got very very lucky that I was able to get the median on two out of the three sides! I'll keep testing and verifying before doing any more posts and pictures so that other members don't waste their time.
Looks good on the list except for:
point 5 and
point 7. Both of them are on line 1 instead of line C.
|
| « Last Edit: Dec 20th, 2003, 9:17pm by Lightboxes » |
IP Logged |
A job is not worth doing unless it's worth doing well.
|
|
|
towr
wu::riddles Moderator
Uberpuzzler
Some people are average, some are just mean.
Gender:
Posts: 13730
|
|
Re: Construct Medians
« Reply #7 on: Dec 21st, 2003, 7:12am » |
Quote Modify
|
If you have the medians of two sides, you can easily find the third, since they all cross in the center of gravity..
|
|
IP Logged |
Wikipedia, Google, Mathworld, Integer sequence DB
|
|
|
Barukh
Uberpuzzler
Gender:
Posts: 2276
|
|
Re: Construct Medians
« Reply #8 on: Dec 21st, 2003, 11:45am » |
Quote Modify
|
on Dec 20th, 2003, 8:37pm, Lightboxes wrote:| I'm glad you have not done any work yet. |
|
Well, actually I did... Instead of making all the nasty calculations, I decided to draw the whole thing using an appropriate software. My choice was MATLAB. I wrote a set of functions, and simulated the case similar to Lightboxes. Unfortunately, the construction was proved to be wrong 
(that's what you also got, right?)
Lightboxes, if you (or somebody else) want to use my work, please let me know, I'll send you the source code.
At the end - the note: in straightedge only constructions, bisecting the given segment is equivalent to drawing a parallel to it (i.e. if we have one, the other may be constructed).
|
|
IP Logged |
|
|
|
Barukh
Uberpuzzler
Gender:
Posts: 2276
|
|
Re: Construct Medians
« Reply #9 on: Dec 26th, 2003, 6:03am » |
Quote Modify
|
OK, I think I've got a solution... After I draw a figure, I will present it here. Meanwhile - some relevant considerations. The formulation of this problem is ... misleading. Namely, it is tempting to think that in order to build any median, one must use the entire configuration. At least, I thought so, and so did Lightboxes. This is wrong.
HINT: given an ellipse and its foci, it is possible to construct the center of the ellipse with straightedge only.
|
|
IP Logged |
|
|
|
Barukh
Uberpuzzler
Gender:
Posts: 2276
|
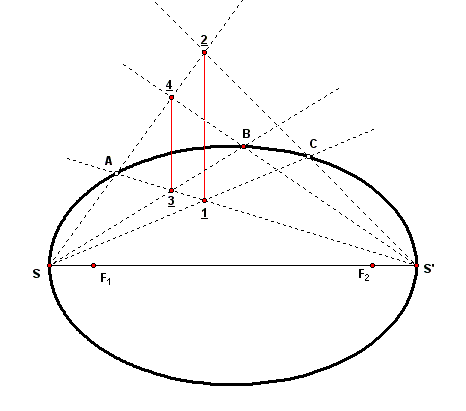
The following is the construction of the center of a given ellipse, if its foci F1 and F2 are also given.
1). Construct the line F1F2 and mark its intersections with the ellipse – points S, S’.
2). Pick three arbitrary points A, B, C on the ellipse (the only restriction – they should not coincide with S and S’). Construct 6 lines joining these points to S and S’.
3). Find the following 4 points of intersection: 1 = SC[smiley=cdot.gif]S’A, 2 = S’C[smiley=cdot.gif]SA; 3 = SB[smiley=cdot.gif]S’A, 4 = S’B[smiley=cdot.gif]SA. Join the points 1 and 2, 3 and 4. What we get is a pair of parallel lines 12 and 34 perpendicular to the SS’ axis. The proof of this statement is omitted (there exists an amazingly simple argument using projective geometry tools).
The steps 1-3 are shown in the attached figure.
4). Using the parallel lines, bisect one of the segments 12 or 34.
5). Using the bisected segment, construct lines parallel to it through points F1 and F2 – call them p1 and p2.
Steps 4-5 are not shown in the figure – let me leave them as an exercise. In fact, they are basic straightedge constructions learned in projective geometry courses. For the interested, I will post the relevant information at this thread also.
6). Lines p1, p2 intersect the ellipse in 4 points that are the vertices of a rectangle. Draw its diagonals – they will intersect in the center of the ellipse.
Happy new year  !
|
| « Last Edit: Dec 27th, 2003, 10:40am by Barukh » |
IP Logged |
|
|
|
Sjoerd Job Postmus
Full Member
Posts: 228
|
|
Re: Construct Medians
« Reply #11 on: Nov 11th, 2005, 3:32am » |
Quote Modify
|
on Dec 27th, 2003, 10:38am, Barukh wrote:The following is the construction of the center of a given ellipse, if its foci F1 and F2 are also given.
1). Construct the line F1F2 and mark its intersections with the ellipse – points S, S’.
2). Pick three arbitrary points A, B, C on the ellipse (the only restriction – they should not coincide with S and S’). Construct 6 lines joining these points to S and S’.
3). Find the following 4 points of intersection: 1 = SC[smiley=cdot.gif]S’A, 2 = S’C[smiley=cdot.gif]SA; 3 = SB[smiley=cdot.gif]S’A, 4 = S’B[smiley=cdot.gif]SA. Join the points 1 and 2, 3 and 4. What we get is a pair of parallel lines 12 and 34 perpendicular to the SS’ axis. The proof of this statement is omitted (there exists an amazingly simple argument using projective geometry tools).
The steps 1-3 are shown in the attached figure.
4). Using the parallel lines, bisect one of the segments 12 or 34.
5). Using the bisected segment, construct lines parallel to it through points F1 and F2 – call them p1 and p2.
Steps 4-5 are not shown in the figure – let me leave them as an exercise. In fact, they are basic straightedge constructions learned in projective geometry courses. For the interested, I will post the relevant information at this thread also.
6). Lines p1, p2 intersect the ellipse in 4 points that are the vertices of a rectangle. Draw its diagonals – they will intersect in the center of the ellipse.
Happy new year ! |
|
Can't we simplify this method?
Draw a line F1F2
Through F1, draw a random line that doesn't go through F2.
Now, draw a parrallel line through F2.
Mark where these lines cross the ellipse.
Construct the diagonals from these 'endpoints'.
Where the diagonals cross, we have the center.
I think this is a more easy way, and I typed a long explanation.
At least, I suspect this is sufficient, and that the lines through F1 and F2 have to be parralel, but don't necesarily have to make a 90* angle with the line F1F2, thus simplifying Barukh's steps, skipping the first few.
|
|
IP Logged |
|
|
|
Barukh
Uberpuzzler
Gender:
Posts: 2276
|
|
Re: Construct Medians
« Reply #12 on: Nov 11th, 2005, 10:40am » |
Quote Modify
|
on Nov 11th, 2005, 3:32am, Sjoerd Job Postmus wrote:
Can't we simplify this method? |
|
Sjoerd, one of the steps in your construction constructs the parallel to an arbitrary line using straightedge alone. How would you do that?
So I am afraid that's not sufficient.
|
|
IP Logged |
|
|
|
SMQ
wu::riddles Moderator
Uberpuzzler
Gender:
Posts: 2084
|
|
Re: Construct Medians
« Reply #13 on: Nov 11th, 2005, 11:23am » |
Quote Modify
|
on Nov 11th, 2005, 10:40am, Barukh wrote:
By running your pencil down the back side of the straightedge, of course. 
--SMQ
|
|
IP Logged |
--SMQ
|
|
|
Zero
Newbie
Posts: 17
|
|
Re: Construct Medians
« Reply #14 on: Jan 14th, 2006, 6:11pm » |
Quote Modify
|
This probably isn't what you folks are looking for so I'm not hiding it. So assuming i have a pencil too... i'd completely ignore the ellipsi and do it the old fashioned way. i.e. high-school method.
a "straight edge" will act just like a standard compass if u make a little dot on it some ways up and rotate it carefully.
-------
another cheesy way of doing it would be keep drawing parallel lines from the ones you have until you got the two tangent points on each ellipse.. join them to bisect the line inside.
----
the most classic cheeseball method involves folding the paper 
there's a few other variants using repetition rather than math.
sometimes u just gotta do what u gotta do to get the job done
|
|
IP Logged |
|
|
|
|
RIDDLES SITE
WRITE MATH!
Home 
Help 
Search 
Members 
Login 
Register
Reply
Notify of replies
Send Topic
Print