 Author
Author |
Topic: Special case Pell equation (Read 2965 times) |
|
Sir Col
Uberpuzzler
    

impudens simia et macrologus profundus fabulae

Gender: 
Posts: 1825
|
 |
Special case Pell equation
« on: Jul 11th, 2008, 2:24am » |
 Quote Quote  Modify Modify
|
Does anyone know if the special case Pell equation, x2 - 2y2 = N, where N is square, has been studied?
Basically I am looking for a general solution to the Diophantine equation:
x2 - 2y2 = k2
Any insights or references would be gratefully received.
|
|
 IP Logged IP Logged |
mathschallenge.net / projecteuler.net
|
|
|
Eigenray
wu::riddles Moderator
Uberpuzzler
    

Gender: 
Posts: 1948
|
 |
Re: Special case Pell equation
« Reply #1 on: Jul 11th, 2008, 9:09am » |
 Quote Quote  Modify Modify
|
I don't know if it's any simpler than the case where N is not a square.
Basically you are looking for all elements x+y 2 2   ( ( 2) which have norm N. Since 2) which have norm N. Since  ( ( 2) is a principal ideal domain, it suffices to find all ideals (x+y 2) is a principal ideal domain, it suffices to find all ideals (x+y 2) of norm N; for each such ideal we have the sequence of solutions 2) of norm N; for each such ideal we have the sequence of solutions  (3+2 (3+2 2)n(x+y 2)n(x+y 2). 2).
For each prime power q || N, we take an element wq of norm q, and then multiply them all together to get an element of norm N. Say q=pa.
For p=2 (p ramified), wq = ( 2)a is the only possibility. 2)a is the only possibility.
For p= 1 mod 8 (p split), there exists z = x+y 1 mod 8 (p split), there exists z = x+y 2 with x2-2y2=p. The other prime ideal of norm p is generated by z' = x-y 2 with x2-2y2=p. The other prime ideal of norm p is generated by z' = x-y 2. So wq = ziz'a-i for some i, 0 2. So wq = ziz'a-i for some i, 0  i i  a. a.
For p= 3 mod 8 (p inert), (p) is prime of norm p2. So wp = pa/2 is the only possibility (there is no solution if a is odd). 3 mod 8 (p inert), (p) is prime of norm p2. So wp = pa/2 is the only possibility (there is no solution if a is odd).
So the total number of ideals is  (1+a), where the product is over all prime powers pa || N where p= (1+a), where the product is over all prime powers pa || N where p= 1 mod 8, and each ideal gives a sequence of solutions by taking all the generators of positive norm. 1 mod 8, and each ideal gives a sequence of solutions by taking all the generators of positive norm.
It is very similar to, say, x2+y2 = N, except there of course the unit group is finite. But the procedure is the same: factor N; for each split prime (here p=1 mod 4) write p as a norm (sum of two squares); then use this to find all ideals of norm N (again  (1+a), where now we run through p=1 mod 4). Then each ideal gives 4 solutions since there are 4 units of norm 1. (1+a), where now we run through p=1 mod 4). Then each ideal gives 4 solutions since there are 4 units of norm 1.
|
| « Last Edit: Jul 11th, 2008, 9:11am by Eigenray » |
 IP Logged IP Logged |
|
|
|
rmsgrey
Uberpuzzler
    

    
Gender: 
Posts: 2874
|
 |
Re: Special case Pell equation
« Reply #2 on: Jul 14th, 2008, 11:25am » |
 Quote Quote  Modify Modify
|
Would it not be simpler to rewrite the equation as:
x2-k2=2y2
|
|
 IP Logged IP Logged |
|
|
|
Eigenray
wu::riddles Moderator
Uberpuzzler
    

Gender: 
Posts: 1948
|
 |
Re: Special case Pell equation
« Reply #3 on: Jul 14th, 2008, 12:05pm » |
 Quote Quote  Modify Modify
|
Ah, I was thinking k was fixed and we wanted to find x and y. We can also give a parameterization for all primitive solutions:
x = u2+2v2
y = 2uv
k =  (u2-2v2) (u2-2v2)
where u,v are relatively prime and u is odd.
|
| « Last Edit: Jul 14th, 2008, 12:08pm by Eigenray » |
 IP Logged IP Logged |
|
|
|
Sir Col
Uberpuzzler
    

impudens simia et macrologus profundus fabulae

Gender: 
Posts: 1825
|
 |
Re: Special case Pell equation
« Reply #4 on: Jul 14th, 2008, 2:37pm » |
 Quote Quote  Modify Modify
|
Thanks for the feedback.
Unfortunately k is fixed. I was looking for a slick parametrisation of the special case Pell equations equations:
x2 - 2y2 = 1
x2 - 2y2 = 4
x2 - 2y2 = 9
et cetera.
I know that Euler dealt with parametrisation of x4 - 2y4 = k2, but I've never seen, what you would expect, the more basic second degree form dealt with. However, my experience and texts are all limited to (basic) elementary number theory and I have little experience with advanced number theory, so it is quite possible that these special cases have been dealt with in more recent years.
|
|
 IP Logged IP Logged |
mathschallenge.net / projecteuler.net
|
|
|
towr
wu::riddles Moderator
Uberpuzzler
    

Some people are average, some are just mean.
Gender: 
Posts: 13730
|
 |
Re: Special case Pell equation
« Reply #5 on: Jul 14th, 2008, 3:17pm » |
 Quote Quote  Modify Modify
|
At first glance, it seems that given the first two solution, you can just apply
x(n+2) = 6x(n+1)-x(n)
y(n+2) = 6y(n+1)-y(n)
to get the rest. (For added slickness, you can find the closed form)
k=1:
3 2
17 12
99 70
577 408
k=2:
6 4
34 24
198 140
1154 816
k=3:
9 6
51 36
297 210
1731 1224
And really, the first series is enough, just multiply by k to get the others. Not sure how to prove this covers all solutions though.
However, if this is all, then it explains why Euler skipped straight to x4 - 2y4 = k2.
|
| « Last Edit: Jul 14th, 2008, 3:20pm by towr » |
 IP Logged IP Logged |
Wikipedia, Google, Mathworld, Integer sequence DB
|
|
|
pex
Uberpuzzler
    

Gender: 
Posts: 880
|
 |
Re: Special case Pell equation
« Reply #6 on: Jul 14th, 2008, 3:46pm » |
 Quote Quote  Modify Modify
|
on Jul 14th, 2008, 3:17pm, towr wrote:| And really, the first series is enough, just multiply by k to get the others. Not sure how to prove this covers all solutions though. |
|
I don't think it does. For k = 7, the smallest solution you'd find by that method would be (x, y) = (21, 14). However, (9, 4) and (11, 6) also work.
Edit: not that I have a better idea...
|
| « Last Edit: Jul 14th, 2008, 3:47pm by pex » |
 IP Logged IP Logged |
|
|
|
Eigenray
wu::riddles Moderator
Uberpuzzler
    

Gender: 
Posts: 1948
|
 |
Re: Special case Pell equation
« Reply #7 on: Jul 14th, 2008, 4:41pm » |
 Quote Quote  Modify Modify
|
Was what I said too abstract? Here is an example: k=3*7*17. We first solve x2-2y2 = p for each prime p dividing N. There is only a solution if p =  1 mod 8 (and this solution is unique up to conjugation and units): 1 mod 8 (and this solution is unique up to conjugation and units):
For p=7, we have (3, 1). 1).
For p=17, we have (5, 2). 2).
Otherwise, we have only the solution (p,0) for x2-2y2=p2.
Now we take our prime solutions and multiply them together to get solutions for N: if (a,b) is a solution for A, and (x,y) is a solution for X, then (a,b)*(x,y) = (ax+2by, ay+bx) is a solution for A*X.
For N=3272172, we can take
(3,0)*(3,1)2*(5,2)2 = (1809, 1254)
(3,0)*(3,1)2*(5,2)(5,-2) = (561, 306)
(3,0)*(3,1)2*(5,-2)2 = (369, -66)
(3,0)*(3,1)(3,-1)*(5,2)2 = (693, 420)
(3,0)*(3,1)(3,-1)*(5,2)(5,-2) = (357, 0)
(3,0)*(3,1)(3,-1)*(5,-2)2 = (693, -420)
(3,0)*(3,-1)2*(5,2)2 = (369, 66)
(3,0)*(3,-1)2*(5,2)(5,-2) = (561, -306)
(3,0)*(3,-1)2*(5,-2)2 = (1809, -1254)
Now any other solution can be obtained from exactly one of these by multiplying by a power of (3,2) (or (3,2)-1=(3,-2)). E.g.,
(3,2)(1809, 1254) = (10443, 7380)
(3,2)-1(1809, 1254) = (411, 144)
[ The solution (x,y) is really the element x+y 2 2   [ [ 2]. An element has norm N iff it generates an ideal of norm N. There are only finitely many such ideals; two elements generates the same ideal iff they differ by a unit, and the group of units with norm 1 is generated by the elements -1 and 3+2 2]. An element has norm N iff it generates an ideal of norm N. There are only finitely many such ideals; two elements generates the same ideal iff they differ by a unit, and the group of units with norm 1 is generated by the elements -1 and 3+2 2. ] 2. ]
Finding the complete set of solutions requires solving congruences x2-2y2=p for each p|k, but I don't see how there could be any way around this.
For practical purposes, the worse part is probably factoring k. Then, for each p, there is a standard algorithm for finding x such that x2=2 mod p. This means that 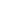 =x+ =x+ 2 has norm divisible by p, so it is divisible by an element 2 has norm divisible by p, so it is divisible by an element  of norm p. Since of norm p. Since  [ [ 2] is a Euclidean domain, we can use the Euclidean algorithm to quickly find 2] is a Euclidean domain, we can use the Euclidean algorithm to quickly find  ~ gcd( ~ gcd(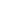 , p). , p).
|
| « Last Edit: Jul 15th, 2008, 6:18am by Eigenray » |
 IP Logged IP Logged |
|
|
|
Eigenray
wu::riddles Moderator
Uberpuzzler
    

Gender: 
Posts: 1948
|
 |
Re: Special case Pell equation
« Reply #8 on: Jul 14th, 2008, 5:18pm » |
 Quote Quote  Modify Modify
|
on Jul 14th, 2008, 2:37pm, Sir Col wrote:| I know that Euler dealt with parametrisation of x4 - 2y4 = k2, but I've never seen, what you would expect, the more basic second degree form dealt with. |
|
Do you know his result? We can start with (3,2,7), and then if (x,y,k) is a solution, so is (x', y', k'), where x' = x4+2y4, y' = 2xyk, k' = 2k4 - x'2. But I'm not sure if this gives this doesn't give all (primitive) solutions.
Key fact: if X2 - 2Y2 = K2 is a primitive solution, then
X = u2+2v2,
Y = 2uv,
K = |u2-2v2|,
where u,v are relatively prime with u odd.
If (x2,y2,k) is a solution, we can assume it is primitive because p|x,y implies p2|k. Taking u,v as above, (x,v,u) is a solution, which is primitive because u,v are relatively prime. Therefore
x = a2+2b2
v = 2ab
u = |a2-2b2|.
Since y2 = 2uv = 4abu is a square, and a,b,u are relatively prime, we know that a=A2, b=B2, and u=U2 are all squares. But now there are two cases.
In the first case, A4-2B4 = U2, i.e., (A,B,U) is a solution which is smaller than the original one [ since a < x unless b=0, a=1, which is the solution (1,0,1) ], and so we are done by induction (with whatever we're proving, not that I know what that is yet).
But in the second case we have A4 - 2B4 = -U2. This leads to
A2 = m2 - n2 + 2mn
B2 = m2 + n2.
We can write (m,n) = (r2-s2, 2rs) and stick that back into the formula for A2, but I don't know if that goes anywhere*.
One solution to the above is (r,s) = (1,0), (A,B,U)=(1,1,1), giving us the solution (x,y,k) = (3,2,7). But there is also the solution (r,s) = (3,2), (m,n) = (5, 12), (A,B,U) = (1, 13, 239), and finally (x,y,k) = (57123, 6214, 3262580153). Similarly, (r,s) = (2,39) gives (A,B,U) = (1343, 1525, 2750257), and (x,y,k) = (14070212996451, 11265465210550, 83545316896178428367654599).
*(Edit): Let C = r2+2rs-s2. Then we have
(C+A)(C-A) = C2 - A2 = 8(rs)2.
Since r,s are relatively prime and of opposite parity, A,C are odd and relatively prime, and gcd(C+A,C-A)=2. If C>0, then {C+A, C-A} = {2f2, 4g2} for integers f, g, with fg=rs, and
r2+2rs-s2 = C = [(C+A)+(C-A)]/2 = f2 + 2g2,
so
2g4-s4 = (gr - fs + gs)2,
meaning (s, g, gr-fs+gs) is a solution, and smaller because g <= rs = n/2 < B, unless f=0, in which case n=0, and A=B=1. Or if C<0, we have
2g4-r4 = (gs - fr - gr)2,
so (r, g, gs-fr-gr) is a smaller solution.
We should be able to work backwards then and obtain all solutions this way.
|
| « Last Edit: Jul 14th, 2008, 9:09pm by Eigenray » |
 IP Logged IP Logged |
|
|
|
towr
wu::riddles Moderator
Uberpuzzler
    

Some people are average, some are just mean.
Gender: 
Posts: 13730
|
 |
Re: Special case Pell equation
« Reply #9 on: Jul 15th, 2008, 1:21am » |
 Quote Quote  Modify Modify
|
on Jul 14th, 2008, 4:41pm, Eigenray wrote:| Was what I said too abstract? |
|
It was a little bit. It's starting to dawn now though.
Quote:Now we take our prime solutions and multiply them together to get solutions for N: if (a,b) is a solution for A, and (x,y) is a solution for X, then (a,b)*(x,y) = (ax+2by, ay+bx) is a solution for A*X.
For N=3272172, we can take
(3,0)*(3,1)2*(5,2)2 = (1809, 1254)
(3,0)*(3,1)2*(5,2)(5,-2) = (561, 306)
(3,0)*(3,1)2*(5,-2)2 = (369, -66)
(3,0)*(3,1)(3,-1)*(5,2)2 = (693, 420)
(3,0)*(3,1)(3,-1)*(5,2)(5,-2) = (357, 0)
(3,0)*(3,1)(3,-1)*(5,-2)2 = (693, -420)
(3,0)*(3,-1)2*(5,2)2 = (369, 66)
(3,0)*(3,-1)2*(5,2)(5,-2) = (561, -306)
(3,0)*(3,-1)2*(5,-2)2 = (1809, -1254)
|
|
Is there a good way to avoid getting both (x,y) and (x,-y) here? Because once we have (x,y), we already know (x,-y), (-x,y) and (-x,-y) are solutions as well.
|
|
 IP Logged IP Logged |
Wikipedia, Google, Mathworld, Integer sequence DB
|
|
|
Eigenray
wu::riddles Moderator
Uberpuzzler
    

Gender: 
Posts: 1948
|
 |
Re: Special case Pell equation
« Reply #10 on: Jul 15th, 2008, 6:13am » |
 Quote Quote  Modify Modify
|
on Jul 15th, 2008, 1:21am, towr wrote:| Is there a good way to avoid getting both (x,y) and (x,-y) here? Because once we have (x,y), we already know (x,-y), (-x,y) and (-x,-y) are solutions as well. |
|
Yes. Note that these symmetries come from two distinct operations: multiplication by -1, which takes (x,y) to (-x,-y), and conjugation, which takes (x,y) to (x,-y). Multiplication by -1 gives the same ideal, which is only counted once. Conjugation, however, usually gives a different ideal. (For the purposes of this problem you can replace 'ideal' with 'element of  [ [ 2] modulo multiplication by units'.) 2] modulo multiplication by units'.)
Say N = 2i pa pa  qb, where each p = qb, where each p =  1 mod 8, and q = 1 mod 8, and q =  3 mod 8. If some b is odd there are no solutions. Otherwise, there are 3 mod 8. If some b is odd there are no solutions. Otherwise, there are  (1+a) 'basic' solutions (ideals), namely: (1+a) 'basic' solutions (ideals), namely:
(0,1)i  zpuzp'a-u zpuzp'a-u  (q,0)b/2. (q,0)b/2.
Conjugating replaces each u with a-u. So we can lexicographically order the (u) vectors and only take the first 'half'. That is, require u1  a1/2; if u1=a1/2, require u2 a1/2; if u1=a1/2, require u2  a2/2; etc. a2/2; etc.
Note that there is an even number of ideals unless all ai are even, in which case N is a square and the odd solution is (N,0), which is the only one preserved by conjugation. (Note that zpzp' = (p,0) by definition.)
|
| « Last Edit: Jul 15th, 2008, 6:27am by Eigenray » |
 IP Logged IP Logged |
|
|
|
|
 WRITE MATH!
WRITE MATH!
 Home
Home  Help
Help  Search
Search  Members
Members  Login
Login  Register
Register WRITE MATH!
WRITE MATH!
 Home
Home  Help
Help  Search
Search  Members
Members  Login
Login  Register
Register