 Author
Author |
Topic: "Nullity" (Read 1238 times) |
|
towr
wu::riddles Moderator
Uberpuzzler
    

Some people are average, some are just mean.
Gender: 
Posts: 13730
|
 |
Re: "Nullity"
« Reply #1 on: Feb 26th, 2007, 1:04pm » |
 Quote Quote  Modify Modify
|
'Nullity' sounds a bit like NaN (in fact, fooling around in javascript a bit; NaN and +/-Infinity seem to behave exactly as laid out in the paper). I had my doubts about it the first time I heard about it, and that hasn't really changed.
What's the added value? Does it actually change anything; other than giving a name to 'undefined value'?
Not to mention, in entropy calculations (for example in computing a decision tree) I'd much prefer have -p log(p) be 0 for p=0 than 'nullity'.
|
| « Last Edit: Feb 26th, 2007, 1:33pm by towr » |
 IP Logged IP Logged |
Wikipedia, Google, Mathworld, Integer sequence DB
|
|
|
SMQ
wu::riddles Moderator
Uberpuzzler
    

Gender: 
Posts: 2084
|
 |
Re: "Nullity"
« Reply #2 on: Feb 26th, 2007, 1:34pm » |
 Quote Quote  Modify Modify
|
on Feb 26th, 2007, 1:04pm, towr wrote:| 'Nullity' sounds a bit like NaN. |
|
Yeah, near as I can tell, their arithmetic rules are exactly what IEEE floats have been using for 20+ years now with the sole exception of only having a positive zero. It sure sounds to me like a few CS profs got together one night down at the pub and said "hey, wouldn't it be smashing if we redefined NaN to be a number, do you think we could get a theory paper and some press out of it? You know, divide by zero and all that, what?"

--SMQ
|
|
 IP Logged IP Logged |
--SMQ
|
|
|
Icarus
wu::riddles Moderator
Uberpuzzler
    

Boldly going where even angels fear to tread.
Gender: 
Posts: 4863
|
 |
Re: "Nullity"
« Reply #3 on: Feb 26th, 2007, 1:49pm » |
 Quote Quote  Modify Modify
|
The main difference between  ("nullity") and NaN is that ("nullity") and NaN is that  = =  while NaN while NaN 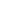 NaN. NaN.
There is also a Wikipedia entry: http://en.wikipedia.org/wiki/James_Anderson_%28computer_scientist%29 that I've found since posting this.
|
| « Last Edit: Feb 26th, 2007, 1:49pm by Icarus » |
 IP Logged IP Logged |
"Pi goes on and on and on ...
And e is just as cursed.
I wonder: Which is larger
When their digits are reversed? " - Anonymous
|
|
|
SMQ
wu::riddles Moderator
Uberpuzzler
    

Gender: 
Posts: 2084
|
 |
Re: "Nullity"
« Reply #4 on: Feb 26th, 2007, 2:01pm » |
 Quote Quote  Modify Modify
|
on Feb 26th, 2007, 1:49pm, Icarus wrote:The main difference between  ("nullity") and NaN is that ("nullity") and NaN is that  = =  while NaN while NaN 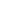 NaN. NaN. |
|
Maybe I'm just out of my depth -- wouldn't be the first time -- but has any one here seen the animated movie "Toy Story"? Remember those little green aliens? Now everybody who does, go "Oooooo" with me. 
Color me still not impressed.
--SMQ
|
|
 IP Logged IP Logged |
--SMQ
|
|
|
towr
wu::riddles Moderator
Uberpuzzler
    

Some people are average, some are just mean.
Gender: 
Posts: 13730
|
 |
Re: "Nullity"
« Reply #5 on: Feb 26th, 2007, 2:36pm » |
 Quote Quote  Modify Modify
|
What do you think about the claims he makes on slide 18 of his sales pitch
Quote:
Fast Computers
Having an arithmetic that works on all combinations of
numbers means I can build computers with:
• No circuitry for handling arithmetical exceptions,
because there are no arithmetical exceptions.
• No circuitry to choose instructions because there is
only one instruction.
• No circuitry to decode an instruction because an
instruction is itself.
This means my computers will run orders of magnitude
faster than today’s computers. |
|
I really don't see how his method would improve on what computers are doing, because just like the IEEE specification he has seperate axioms for his non-real numbers. Which means they'll all be exceptions to the rules applied to regular numbers.
|
|
 IP Logged IP Logged |
Wikipedia, Google, Mathworld, Integer sequence DB
|
|
|
Grimbal
wu::riddles Moderator
Uberpuzzler
    

I am sorry Dave, I *can* do that.
Gender: 
Posts: 7528
|
 |
Re: "Nullity"
« Reply #6 on: Feb 26th, 2007, 3:20pm » |
 Quote Quote  Modify Modify
|
But if  = =  then certainly then certainly  - -  = 0. = 0.
And we can compute  = 0/0 = (0-0)/0 = 0/0 - 0/0 = = 0/0 = (0-0)/0 = 0/0 - 0/0 =  - -  = 0. = 0.
|
|
 IP Logged IP Logged |
|
|
|
towr
wu::riddles Moderator
Uberpuzzler
    

Some people are average, some are just mean.
Gender: 
Posts: 13730
|
 |
Re: "Nullity"
« Reply #7 on: Feb 26th, 2007, 3:23pm » |
 Quote Quote  Modify Modify
|
on Feb 26th, 2007, 3:20pm, Grimbal wrote:But if  = =  then certainly then certainly  - -  = 0. = 0.
And we can compute  = 0/0 = (0-0)/0 = 0/0 - 0/0 = = 0/0 = (0-0)/0 = 0/0 - 0/0 =  - -  = 0. = 0.
|
|
Heh, and he dared claim NaN 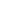 NaN made the computer arithmatic invalid NaN made the computer arithmatic invalid 
|
|
 IP Logged IP Logged |
Wikipedia, Google, Mathworld, Integer sequence DB
|
|
|
Icarus
wu::riddles Moderator
Uberpuzzler
    

Boldly going where even angels fear to tread.
Gender: 
Posts: 4863
|
 |
Re: "Nullity"
« Reply #8 on: Feb 26th, 2007, 3:31pm » |
 Quote Quote  Modify Modify
|
Actually, in his arithmetic, a - a = 0 holds only for Real a.  - -  = =  - -  = =  . .
He claims that his theory has been tested and is consistent, and I have reason to believe this - though I don't buy that his machine testing is adequate (I may be wrong about that, though).
|
|
 IP Logged IP Logged |
"Pi goes on and on and on ...
And e is just as cursed.
I wonder: Which is larger
When their digits are reversed? " - Anonymous
|
|
|
Sir Col
Uberpuzzler
    

impudens simia et macrologus profundus fabulae

Gender: 
Posts: 1825
|
 |
Re: "Nullity"
« Reply #9 on: Feb 27th, 2007, 12:28am » |
 Quote Quote  Modify Modify
|
I'm probably completely out of my depth here, but how does his extended system handle limits?
As x->0+, 1/x->+inf.
As x->0-, 1/x->-inf.
So can he just axiomatically state that 1/0+ = 1/0- = +inf and ignore evidence of limits?
|
| « Last Edit: Feb 27th, 2007, 10:21am by Sir Col » |
 IP Logged IP Logged |
mathschallenge.net / projecteuler.net
|
|
|
Icarus
wu::riddles Moderator
Uberpuzzler
    

Boldly going where even angels fear to tread.
Gender: 
Posts: 4863
|
 |
Re: "Nullity"
« Reply #10 on: Feb 27th, 2007, 5:34am » |
 Quote Quote  Modify Modify
|
Actually, his approach to that is quite kosher: all that shows is that f(x) = 1/x is not continuous at 0.
|
|
 IP Logged IP Logged |
"Pi goes on and on and on ...
And e is just as cursed.
I wonder: Which is larger
When their digits are reversed? " - Anonymous
|
|
|
Sir Col
Uberpuzzler
    

impudens simia et macrologus profundus fabulae

Gender: 
Posts: 1825
|
 |
Re: "Nullity"
« Reply #11 on: Feb 27th, 2007, 10:12am » |
 Quote Quote  Modify Modify
|
But how does he answer the question as x approaches zero from each side that 1/x has different limits? Surely the axiom that 1/0 = +inf undermines fundamental principles in calculus.
Also, if nullity is a number, where does it exist? To just claim it is a number and write it above zero on the number line is hardly answering that question.
And what about... ?
xn – 1 = (x – 1)(xn–1 + xn–2 + ... + x2 + x + 1)
Therefore (xn – 1)/(x - 1) = xn–1 + xn–2 + ... + x2 + x + 1
If we let x = 1, (xn – 1)/(x - 1) = 0/0, but xn–1 + xn–2 + ... + x2 + x + 1 = n.
In other words, it can be shown that 0/0 is equal to any positive whole number n. Hence stating that 0/0 = nullity suggests that nullity is any positive whole number you want.
|
| « Last Edit: Feb 27th, 2007, 10:17am by Sir Col » |
 IP Logged IP Logged |
mathschallenge.net / projecteuler.net
|
|
|
towr
wu::riddles Moderator
Uberpuzzler
    

Some people are average, some are just mean.
Gender: 
Posts: 13730
|
 |
Re: "Nullity"
« Reply #12 on: Feb 27th, 2007, 2:01pm » |
 Quote Quote  Modify Modify
|
on Feb 27th, 2007, 10:12am, Sir Col wrote:| In other words, it can be shown that 0/0 is equal to any positive whole number n. |
|
Only IF you can divide by 0 like you can divide by any non-zero number, but that's the whole problem.
In normal arithmatic you can onyl do that trick when x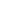 1 1
|
|
 IP Logged IP Logged |
Wikipedia, Google, Mathworld, Integer sequence DB
|
|
|
Icarus
wu::riddles Moderator
Uberpuzzler
    

Boldly going where even angels fear to tread.
Gender: 
Posts: 4863
|
 |
Re: "Nullity"
« Reply #13 on: Feb 27th, 2007, 9:27pm » |
 Quote Quote  Modify Modify
|
on Feb 27th, 2007, 10:12am, Sir Col wrote:But how does he answer the question as x approaches zero from each side that 1/x has different limits? Surely the axiom that 1/0 = +inf undermines fundamental principles in calculus.
Also, if nullity is a number, where does it exist? To just claim it is a number and write it above zero on the number line is hardly answering that question.
And what about... ?
xn – 1 = (x – 1)(xn–1 + xn–2 + ... + x2 + x + 1)
Therefore (xn – 1)/(x - 1) = xn–1 + xn–2 + ... + x2 + x + 1
If we let x = 1, (xn – 1)/(x - 1) = 0/0, but xn–1 + xn–2 + ... + x2 + x + 1 = n.
In other words, it can be shown that 0/0 is equal to any positive whole number n. Hence stating that 0/0 = nullity suggests that nullity is any positive whole number you want. |
|
Let f(x) = x/|x| if x 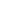 0, f(0) = 0. This is a well-known step function. Note that f(0-) = -1, f(0+) = 1, and yet f has a value at 0 which is neither one. A discontinuous function does not have to have the same limit from both sides. It's value does not have to match either of the limits or have any relation to the limits what-so-ever. It is exactly that lack of a relationship that makes the function discontinuous. The same comments hold true for f(x) = 1/x with his definition. The value of f(0) is not required in any way to match that of f(0+) or f(0-). 0, f(0) = 0. This is a well-known step function. Note that f(0-) = -1, f(0+) = 1, and yet f has a value at 0 which is neither one. A discontinuous function does not have to have the same limit from both sides. It's value does not have to match either of the limits or have any relation to the limits what-so-ever. It is exactly that lack of a relationship that makes the function discontinuous. The same comments hold true for f(x) = 1/x with his definition. The value of f(0) is not required in any way to match that of f(0+) or f(0-).
As for the other calculation, you can't get from the first equation to the second equation when x=1 under his rules. If this seems like a problem, then let me remind you that you can't do that under the normal rules of arithmetic either.
He definitions are misleading in places, and  = 0-1 is one of these, because he actually defines = 0-1 is one of these, because he actually defines  * 0 = * 0 =  . In his system 0 still has no true inverse, and neither does . In his system 0 still has no true inverse, and neither does  . .
________________________________________
Of the various complaints he has gotten, almost all can be divided up into 4 groups:
- "You can't do that!" - the large majority of responses are from people who are incensed by the very idea of doing something beyond what they have learned. These are the same sort of people who get incensed by the suggestion that 0.999... = 1. Their complaints are caused by their own mathematical naivety, not by any fallacy in the argument. They usually cannot even describe their complaint. They "just know this doesn't work".
- "That's contradictory" - these are complaints like Grimbal's and Sir Col's above. They find particular calculations that appear to give contradictory results. Without fail, these contradictions turn out to be the result of calculating "intuitively" rather than by rigidly following his rules. Part of this is due to his desire to make every basic math calculation performable in all cases. Therefore he has to have a value for 1/0, even though 0 has no inverse in his system. (It does have a "reciprocal" - a word he defines to be different in meaning from "inverse". The product of a number & its reciprocal can be either 1 or
 .) He says his system is consistent, and I have every reason to believe this. Though I have not bothered to do so fully, all that is required to show consistency is to build a model of his theory within one that is already considered consistent. This is not hard to do (though his apparent inability to distinguish between definitions and axioms make it harder). .) He says his system is consistent, and I have every reason to believe this. Though I have not bothered to do so fully, all that is required to show consistency is to build a model of his theory within one that is already considered consistent. This is not hard to do (though his apparent inability to distinguish between definitions and axioms make it harder).
- "Where or what is
 " - this objection simply does not hold water. There is no requirement that a new number must somehow be given a "position" relative to the current number line. And it is quite easy to construct a new object which you can use to model " - this objection simply does not hold water. There is no requirement that a new number must somehow be given a "position" relative to the current number line. And it is quite easy to construct a new object which you can use to model  . .
- "This isn't new and it isn't useful" - here is the objection that holds true. You may note that while I have been refuting consistency arguments, I never said anything about towr's doubt of the effectiveness of this system. While his
 is not exactly the same as NaN, it isn't new. I played around with the exact same idea in college, and I have no doubt whatsoever that other mathematicians have explored it as well. But we all dropped it, because it was fairly obvious that there was no mathematical advantage in making the definition. Indeterminate forms remain indeterminate under his system. Just because he gives 0/0 a particular value does not change the fact that 0/0 limits can take on all sorts of values. In fact, rather than decreasing the number of exceptions we must make in our equations, it increases them. For example, Sir Col's formula: (xn - 1)/(x - 1) = xn-1 + ... + 1. Normally when stating this, we do not need to mention that it does not hold for x = 1, since it is clearly undefined there. With nullity around, it is not undefined, but is still unequal, so we must explicitly exclude 1 from the values of x. A more dire objection comes from the common expression of x0 as the constant term of a polynomial or power series. In his formulation, 00 = is not exactly the same as NaN, it isn't new. I played around with the exact same idea in college, and I have no doubt whatsoever that other mathematicians have explored it as well. But we all dropped it, because it was fairly obvious that there was no mathematical advantage in making the definition. Indeterminate forms remain indeterminate under his system. Just because he gives 0/0 a particular value does not change the fact that 0/0 limits can take on all sorts of values. In fact, rather than decreasing the number of exceptions we must make in our equations, it increases them. For example, Sir Col's formula: (xn - 1)/(x - 1) = xn-1 + ... + 1. Normally when stating this, we do not need to mention that it does not hold for x = 1, since it is clearly undefined there. With nullity around, it is not undefined, but is still unequal, so we must explicitly exclude 1 from the values of x. A more dire objection comes from the common expression of x0 as the constant term of a polynomial or power series. In his formulation, 00 =  , not 1, so with every power series in sigma notation, an exception must be made for x = 0. It also causes problems with such functions as (sin x)/x. This function is analytic, provided one removes the removable singularity at 0. But with his tranreals, we would have to come up with another notation for the function (which admittedly there is for this function - but not for other examples) to indicate that at x = 0, we want the value 1, not the defined value of , not 1, so with every power series in sigma notation, an exception must be made for x = 0. It also causes problems with such functions as (sin x)/x. This function is analytic, provided one removes the removable singularity at 0. But with his tranreals, we would have to come up with another notation for the function (which admittedly there is for this function - but not for other examples) to indicate that at x = 0, we want the value 1, not the defined value of  . .
In the end, the downfall of transreals is that they bring nothing of real value to the mathematical table. As for the computational table for which they were designed, I can't speak with authority, but I too fail to see how they would eliminate exceptions, as the non-real transreals are produced by exceptions to the ordinary calculation rules.
|
|
 IP Logged IP Logged |
"Pi goes on and on and on ...
And e is just as cursed.
I wonder: Which is larger
When their digits are reversed? " - Anonymous
|
|
|
Grimbal
wu::riddles Moderator
Uberpuzzler
    

I am sorry Dave, I *can* do that.
Gender: 
Posts: 7528
|
 |
Re: "Nullity"
« Reply #14 on: Feb 28th, 2007, 2:09am » |
 Quote Quote  Modify Modify
|
on Feb 27th, 2007, 9:27pm, Icarus wrote:
- "You can't do that!" - the large majority of responses are from people who are incensed by the very idea of doing something beyond what they have learned. These are the same sort of people who get incensed by the suggestion that 0.999... = 1. Their complaints are caused by their own mathematical naivety, not by any fallacy in the argument. They usually cannot even describe their complaint. They "just know this doesn't work".
|
|
It really sounds like you are saying that if we don't agree we are just as stupid as those who think 0.999... < 1.
When he says his arithmetic is consistent, It is only as long as he doesn't say it isn't. I'm not really convinced when I see:
[A8] a-a = 0 (if a <> inf, -inf, phi)
[A18] a/a = 1 (if a <> 0, inf, -inf, phi)
and also
[A19] (a-1)-1 = a (if a<>-inf)
It shows these extra "numbers" don't work well for algebra. Division by zero is a special case and whatever you do with it remains a special case. In this sense it doesn't "solve the problem of 0/0". The new arithmetic won't take care of it for you.
However this kind of arithmetic is useful in a program to be able to return a result to an otherwise invalid operation. It lets you do calculations without bothering too much about the validity of the inputs and check only at the end whether the result is NaN which would indicate a problem somewhere in the calculations.
So, OK for arithmetics but not for algebra.
[edit] embarassing typo [/edit]
|
| « Last Edit: Mar 6th, 2007, 8:34am by Grimbal » |
 IP Logged IP Logged |
|
|
|
JiNbOtAk
Uberpuzzler
    

Hana Hana No Mi
Gender: 
Posts: 1187
|
 |
Re: "Nullity"
« Reply #15 on: Feb 28th, 2007, 4:55pm » |
 Quote Quote  Modify Modify
|
on Feb 28th, 2007, 2:09am, Grimbal wrote:
It really sounds like you are saying that if we don't agree we are just as stupid as those who think 0.999 < 1.
|
|
But, Grimbal, 0.999 is lesser than 1. 
|
|
 IP Logged IP Logged |
Quis custodiet ipsos custodes?
|
|
|
Icarus
wu::riddles Moderator
Uberpuzzler
    

Boldly going where even angels fear to tread.
Gender: 
Posts: 4863
|
 |
Re: "Nullity"
« Reply #16 on: Feb 28th, 2007, 6:34pm » |
 Quote Quote  Modify Modify
|
on Feb 28th, 2007, 2:09am, Grimbal wrote:| It really sounds like you are saying that if we don't agree we are just as stupid as those who think 0.999 < 1. |
|
There are 4 classes of arguments listed there. Only the first class do I dismiss as foolish. And that is because these are the people who reject the idea without bothering to understand it, or having any solid basis (right or wrong) for their objections.
The second and third class, I hold as mistaken, but their error is a much more understandable one. They are either mislead by a confusing symbolism into believing the theory says things that it actually doesn't (the inconsistency arguments), or they have a weakness in their own understanding of how mathematics works that causes them to feel the approach defies logic (the "where is it?" argument). Both of these people are able to articulate their objections, giving at least a semi-solid foundation for them.
(By the way, I do not consider someone who says 0.999... < 1 to be stupid unless they cling to that position regardless of all evidence. Until then they are just suffering from a misconception. Since I also regularly suffer misconceptions, I find it impossible to judge this harshly.)
And as I indicated in the previous post, the fourth class of class of argument is one I wholeheartedly agree with!
As for the rest of your post, I don't accept that his arithmetic is consistent because he says it is. In fact what comments he made about how he has shown consistency do not convince me at all. I am willing to accept that his theory is consistent because I am well enough aware of the issues to know that it is entirely possible to construct a consistent theory that follows the general shape of this one. At worst, if his theory actually is inconsistent, then adjusting a few axioms would solve the problem. I am willing to accept that he has found the axioms that work, without going through the steps that would explicitly show it.
All of your comments after "I'm not really convinced when I see:" do not address the issue of consistency at all. Instead, they are all about how useful the theory is, and in that, they echo the same concerns that towr has expressed, and I have echoed. Note the closing sentences of my post:
on Feb 27th, 2007, 9:27pm, Icarus wrote:| In the end, the downfall of transreals is that they bring nothing of real value to the mathematical table. As for the computational table for which they were designed, I can't speak with authority, but I too fail to see how they would eliminate exceptions, as the non-real transreals are produced by exceptions to the ordinary calculation rules. |
|
|
|
 IP Logged IP Logged |
"Pi goes on and on and on ...
And e is just as cursed.
I wonder: Which is larger
When their digits are reversed? " - Anonymous
|
|
|
|
 WRITE MATH!
WRITE MATH!
 Home
Home  Help
Help  Search
Search  Members
Members  Login
Login  Register
Register WRITE MATH!
WRITE MATH!
 Home
Home  Help
Help  Search
Search  Members
Members  Login
Login  Register
Register